Question 1.
Solve the following differential equations:
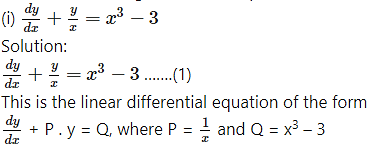
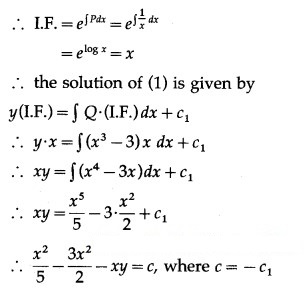
This is the general solution.
Solution:

Solution: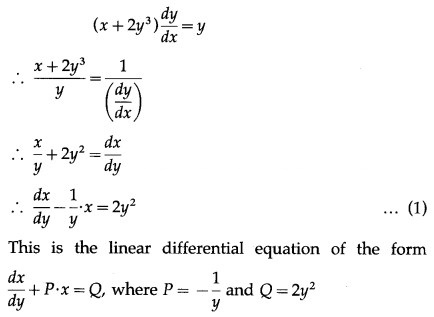


Solution:

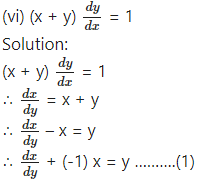
This is the linear differential equation of the form
This is the general solution.
Solution: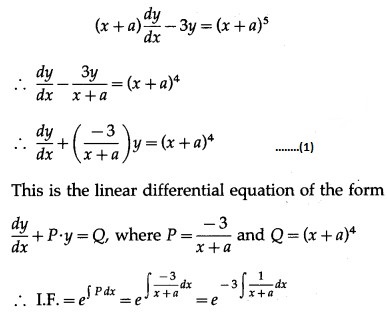

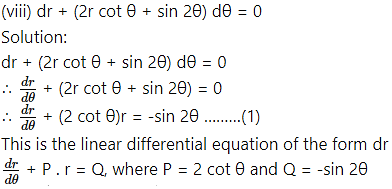
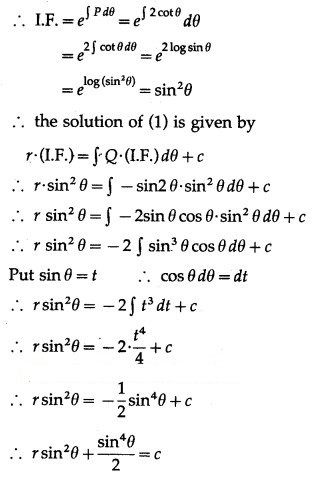
This is the general solution.

This is the linear differential equation of the form
This is the general solution.
Solution:
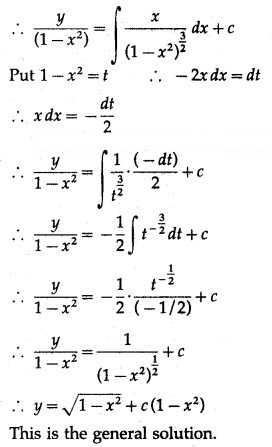
Solution: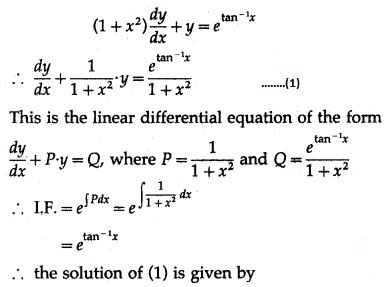

Question 2.
Find the equation of the curve which passes through the origin and has the slope x + 3y – 1 at any point (x, y) on it.
Solution:
Let A(x, y) be the point on the curve y = f(x).
This is the linear differential equation of the form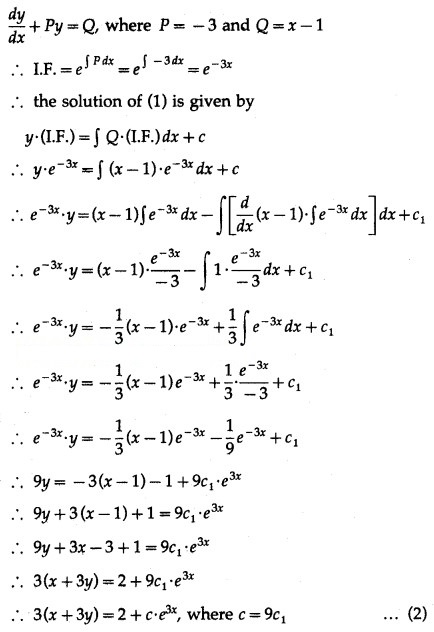
This is the general equation of the curve.
But the required curve is passing through the origin (0, 0).
∴ by putting x = 0 and y = 0 in (2), we get
0 = 2 + c
∴ c = -2
∴ from (2), the equation of the required curve is 3(x + 3y) = 2 – 2 i.e. 3(x + 3y) = 2 (1 – ).
Question 4.
The curve passes through the point (0, 2). The sum of the coordinates of any point on the curve exceeds the slope of the tangent to the curve at any point by 5. Find the equation of the curve.
Solution:
∴ the solution of (1) is given by
This is the general equation of the curve.
But the required curve is passing through the point (0, 2).
∴ by putting x = 0, y = 2 in (2), we get
2 = 4 – 0 + c
∴ c = -2
∴ from (2), the equation of the required curve is y = 4 – x – 2.
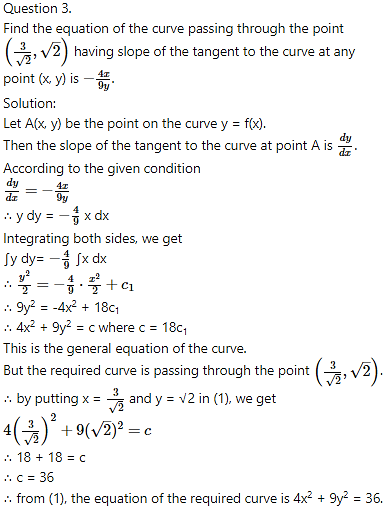
Question 5.
If the slope of the tangent to the curve at each of its point is equal to the sum of abscissa and the product of the abscissa and ordinate of the point. Also, the curve passes through the point (0, 1). Find the equation of the curve.
Solution:
∴ the solution of (1) is given by
y . (I.F.) = ∫Q . (I.F.) dx + c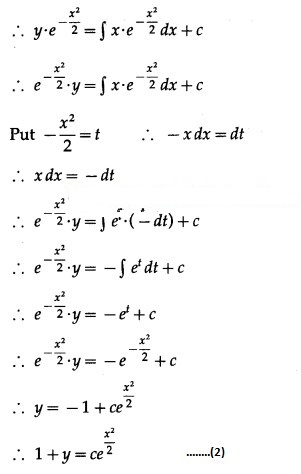
This is the general equation of the curve.
But the required curve is passing through the point (0, 1).
∴ by putting x = 0 and y = 1 in (2), we get
1 + 1 = c
∴ c = 2
∴ from (2), the equation of the required curve is 1 + y = 2.
