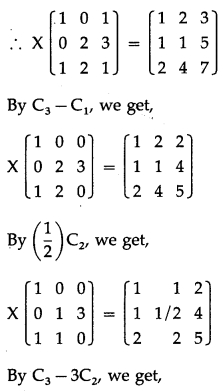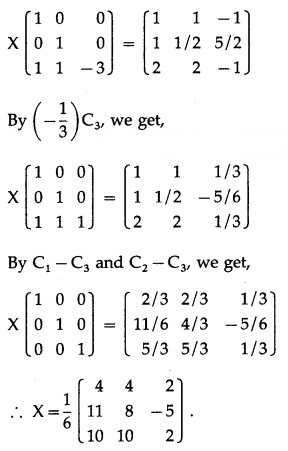Question 1.
Question 2.
By R1 – R2, we get,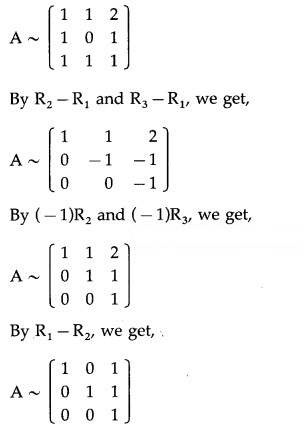
By R1 – R3 and By R2 – R3, we get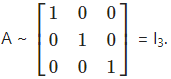
Question 3.
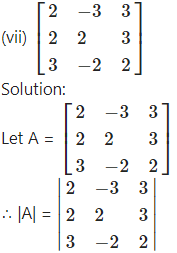
Check whether the following matrices are invertible or not:
∴ A is a non-singular matrix.
Hence, A-1 exist.
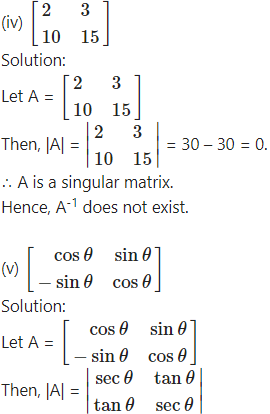
= sec2θ – tan2θ = 1 ≠ 0.
∴ A is a non-singular matrix.
Hence, A-1 exist.
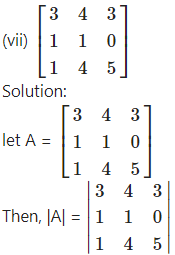
= 3(5 – 0) – 4(5 – 0) + 3(4 – 1)
= 15 – 20 + 9 = 4 ≠ 0
∴ A is a non-singular matrix.
Hence, A-1 exist.
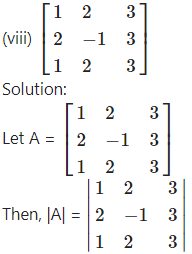
= 1 (-3 -6) – 2 (6 – 3) + 3 (4 + 1)
= -9 – 6 + 15 = 0
∴ A is a singular matrix.
Hence, A-1 does not exist.

= 1(32 – 30) – 2(24 – 20) + 3(18 – 16)
= 2 – 8 + 6 = 0
∴ A is a singular matrix.
Hence, A-1 does not exist.
Question 4.
Solution: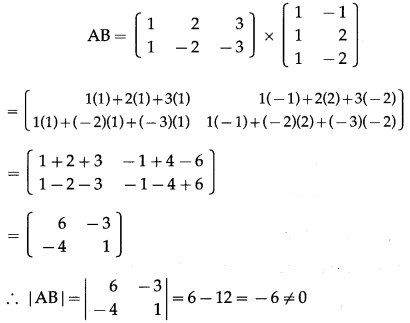
∴ A is a non-singular matrix.
Hence, (AB)-1 exist.
Question 5.
Solution:
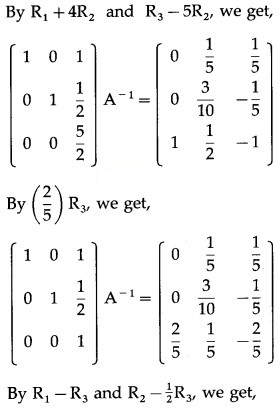
Since A is a non-singular matrix, then find A-1 by using elementary row transformations.
We write AA-1 = I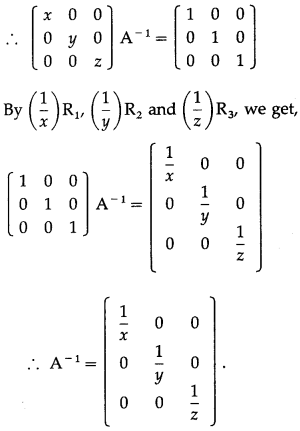
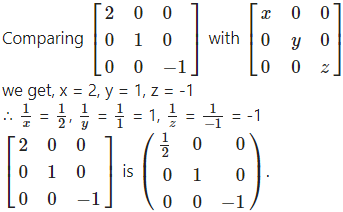
Question 6.
Solution:
We will reduce the matrix A to the identity matrix by using row transformations. During this pro¬cess, I will be converted to the matrix X.
We have AX = I.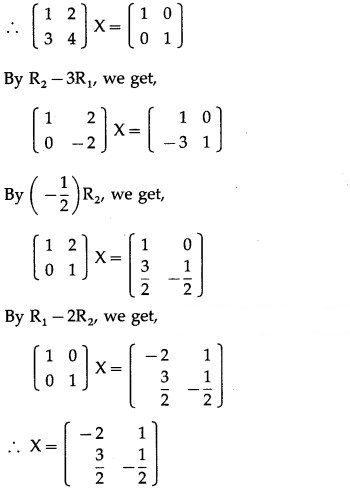
Question 7.
Find the inverse of each of the following matrices (if they exist).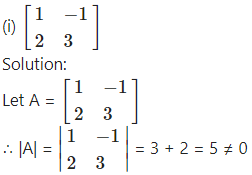
∴ A-1 exists.
Consider AA-1 = I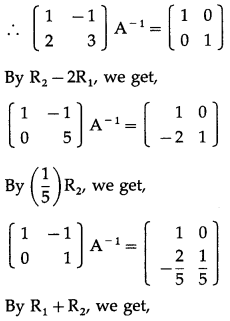
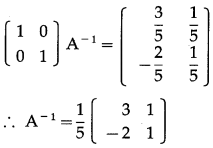
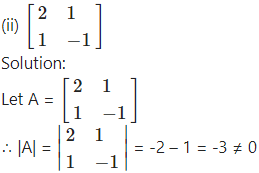
∴ A-1 exists.
Consider AA-1 = I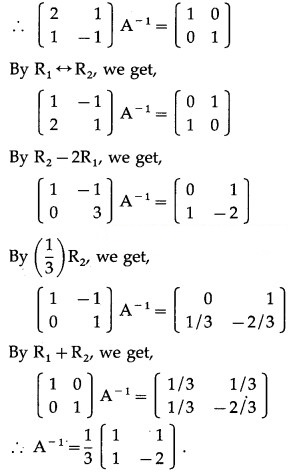
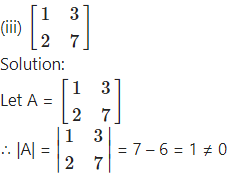
∴ A-1 exists.
Consider AA-1 = I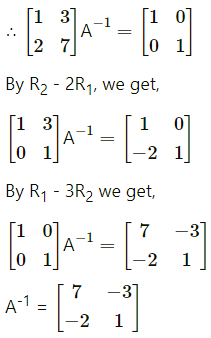

∴ A-1 exists.
Consider AA-1 = I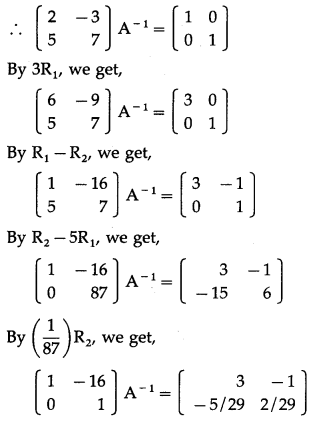
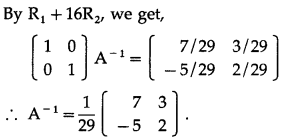
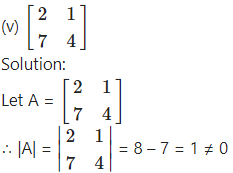
∴ A-1 exists.
Consider AA-1 = I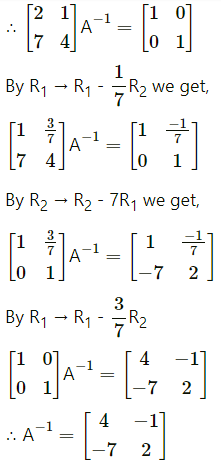

∴ A-1 exists.
Consider AA-1 = I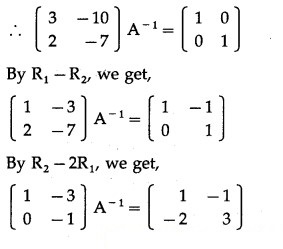
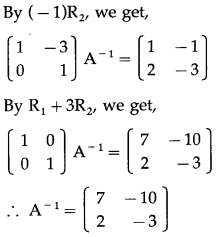
= 2(4 + 6) +3(4 – 9) + 3(-4 – 6)
= 20 – 15 – 30 = -25 ≠ 0
∴ A-1 exists.
Consider AA-1 = I
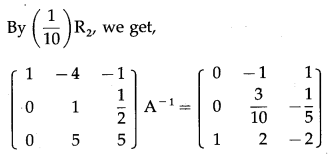
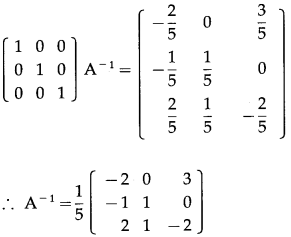

= 1(0 + 25) + 3(0 + 10) + 2(-15 – 0)
= 25 + 30 -30
= 25 ≠ 0
∴ A-1 exists.
Consider AA-1 = I

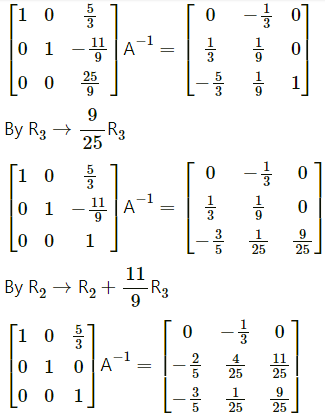
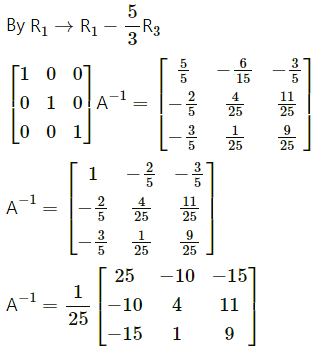
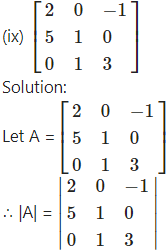
= 2(3 – 0) – 0 – 1(5 – 0)
= 6 – 0 – 5 = 1 ≠ 0
∴ A-1 exists.
Consider AA-1 = I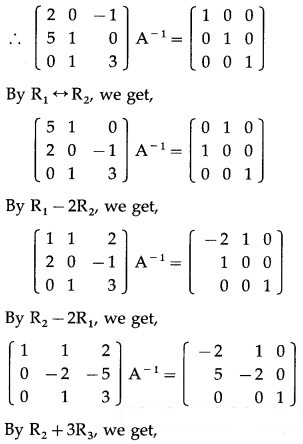
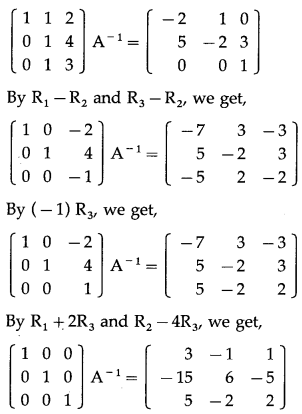
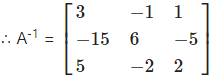
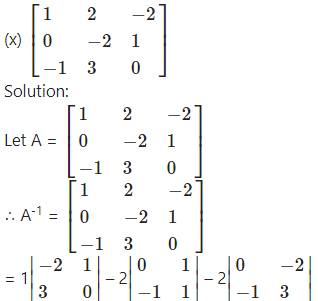
|A| = 1(0 – 3) – 2(0 + 1) – 2(0 – 2)
= -3 – 2 + 4
= -1 ≠ 0
∴ A-1 exists.
We have
AA-1 = I
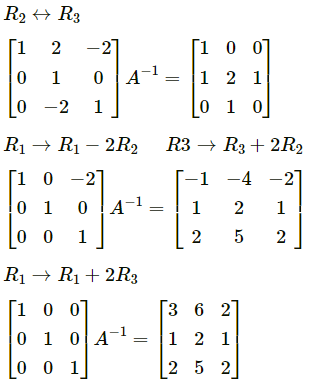

Question 8.
= cosθ (cosθ – 0) + sinθ (sinθ – 0) + 0
= cos2θ + sin2θ = 1 ≠ 0
∴ A-1 exists.
(i) Consider AA-1 = I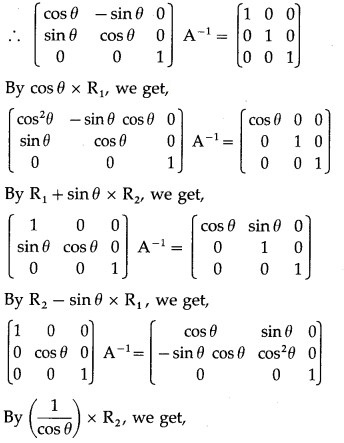
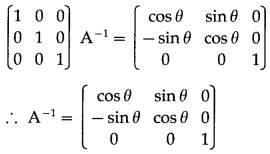
(ii) elementary column transformations
Solution:
Consider A-1A = I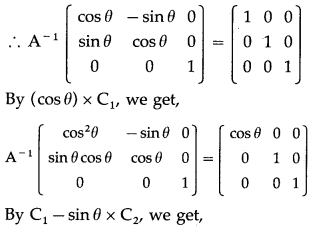
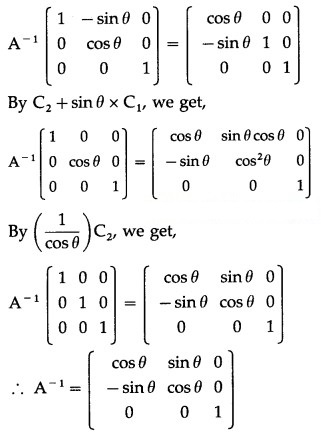
Question 9.
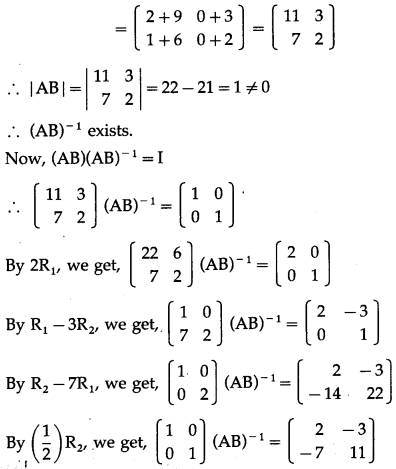
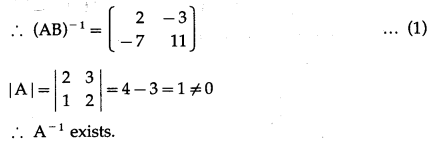
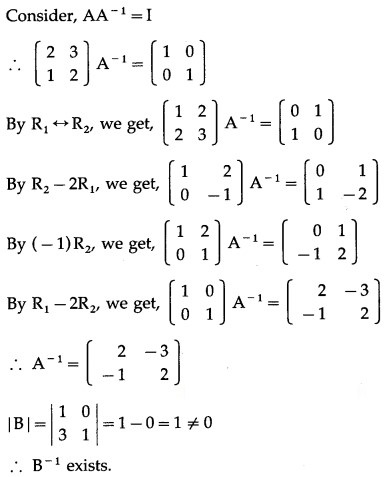
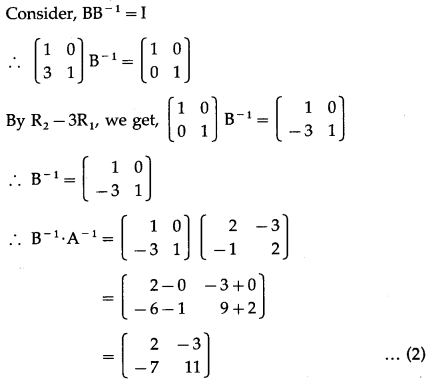
From (1) and (2), (AB)-1 = B-1 ∙ A-1.
Question 10.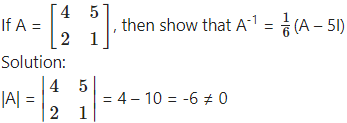
∴ A-1 exists.
Consider AA-1 = I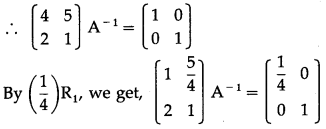
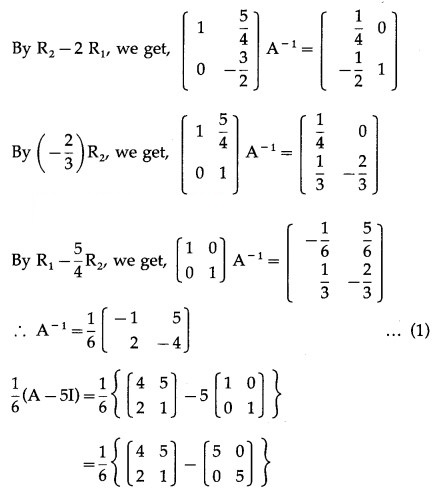
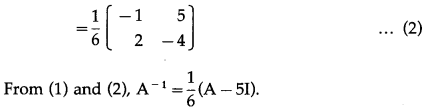
Question 11.
Solution:
AX = B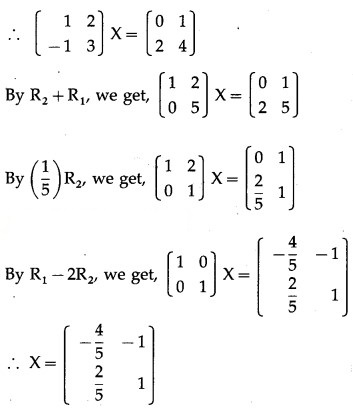
Question 12.
Solution:
AX = B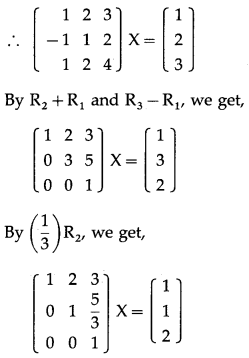
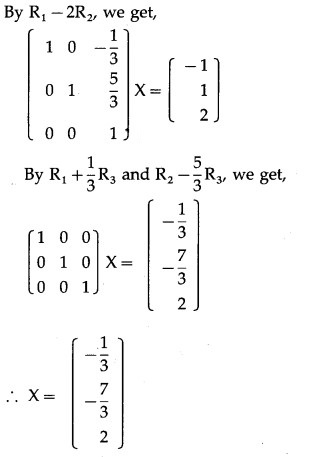
Question 13.
First we perform the row transformations.
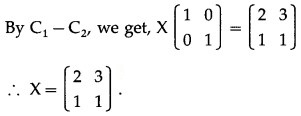
Question 14.
= 1(7 – 20) – 2(7 – 10) + 3(4 – 2)
= -13 + 6 + 6 = -1 ≠ 0
∴ A-1 exists.
First we have to find the cofactor matrix
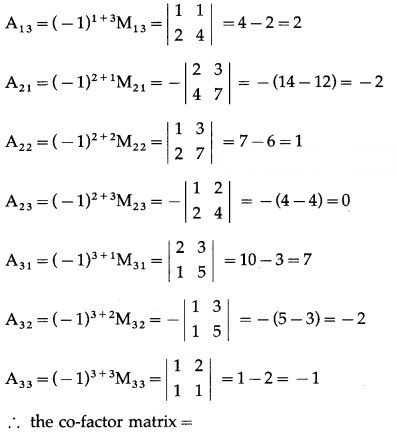
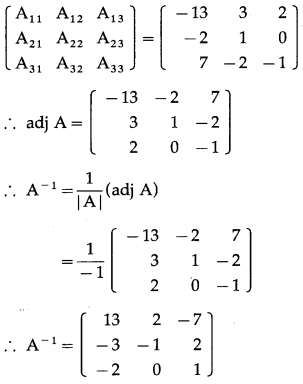
Question 15.
|A| = 1(2 – 6) – 0(0 – 3) + 1(0 – 2)
|A| = -4 – 2
|A| = -6 ≠ 0
∴ A-1 exists.
First we have to find the cofactor matrix
= [Aij]3×3, where Aij = (-1)i+jMij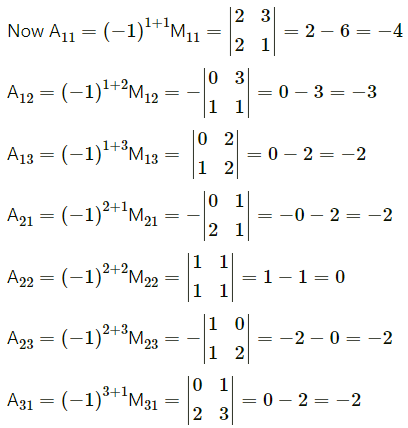
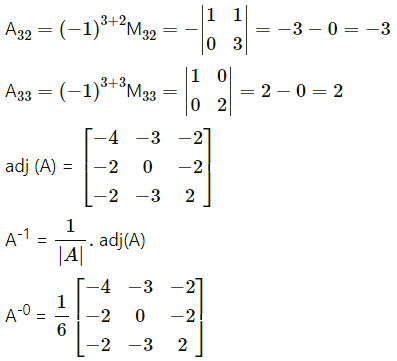
Question 16.
= 1(4 – 4) – 2(-4 – 2) + 3(-2 – 1)
= 0 + 12 – 9 = 3 ≠ 0
∴ A-1 exists.
A-1by adjoint method :
We have to find the cofactor matrix
= [Aij]3×3, where Aij = (-1)i+j Mij
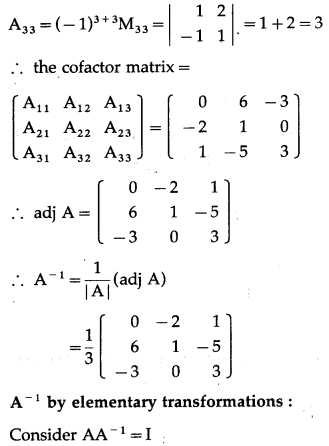
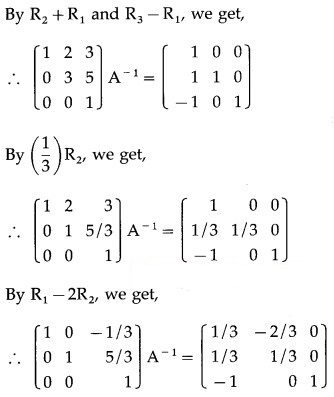

Question 17.
= 1 (2 – 6) – 0 + 1 (0 – 2)
= -4 – 2= -6 ≠ 0
∴ A-1 exists.
Consider A-1A = I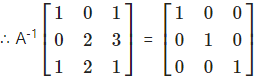
By C3 – C1, we get,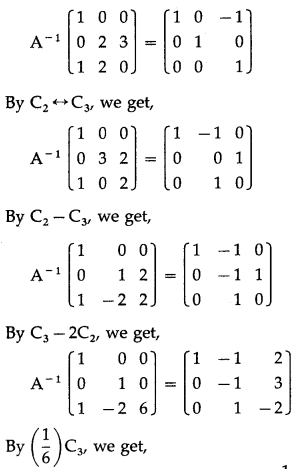
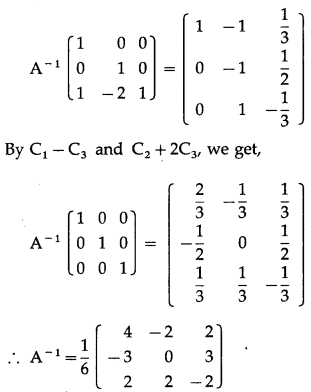
Question 18.
= 1(7 – 20) – 2(7 – 10) + 3(4 – 2)
= -13 + 6 + 6 = -1 ≠ 0
∴ A-1 exists.
Consider AA-1 = I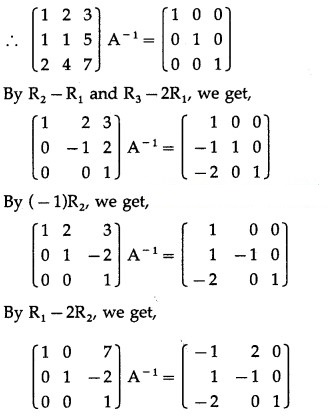

Question 19.
Show with usual notations that for any matrix A = [aij]3×3
(i) a11A21 + a12A22 + a13A23 = 0
Solution: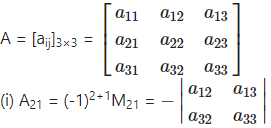
= -(a12a33 – a13a32)
= -a12a33 + a13a32![]()
= a11a33 – a13a31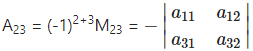
= -(a11a32 – a12a31)
= -a11a32+ a12a31
∴ a11A21 + a12A22 + a13A23
= a11(-a1233 + a13a32) + a12(a11a33 – a13a31) + a13(-a11a32 + a12a31)
= -a11a12a33 + a11a13a32 + a11a12a33 – a12a13a31 – a11a13a32 + a12a13a31
= 0
(ii) a11A11 + a12A12 + a13A13 = |A|
Solution: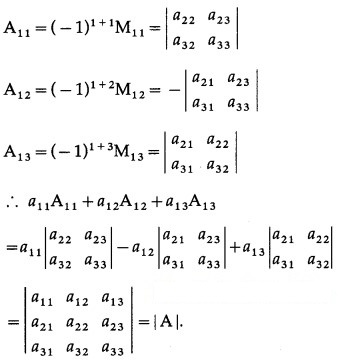
Question 20.
Solution:
Consider XA = B