Question 1.
Choose the correct alternative answer for the questions given below. [1 Mark each]
i. Which one of the following is an irrational number?
Answer:
√5
ii. Which of the following is an irrational number?
iii. Decimal expansion of which of the following is non-terminating recurring?
Answer:
(C) 3/11
iv. Every point on the number line represents which of the following numbers?
(A) Natural numbers
(B) Irrational numbers
(C) Rational numbers
(D) Real numbers
Answer:
(D) Real numbers
Answer:
(A) 4/9
vi. What is √n , if n is not a perfect square number ?
(A) Natural number
(B) Rational number
(C) Irrational number
(D) Options A, B, C all are correct.
Answer:
(C) Irrational number
vii. Which of the following is not a surd ?
Answer:![]()
(A) 3
(B) 2
(C) 6
(D) 5
Answer:
(C) 6
ix. Which one is the conjugate pair of 2√5 + √3 ?
(A) -2√5 + √3
(B) -2√5 – √3
(C) 2√3 – √5
(D) √3 + 2√5
Answer:
(A) -2√5 + √3
x. The value of |12 – (13 + 7) x 4| is ____ .
(A) – 68
(B) 68
(C) – 32
(D) 32
Answer:
(B) 68
Hints:
ii. Since the decimal expansion is neither terminating nor recurring, 0.101001000…. is an irrational number.

v. Let x = [/latex]0.\dot { 4 }[/latex]
∴10 x = [/latex]0.\dot { 4 }[/latex]
∴10 – x = [/latex]4.\dot { 4 }[/latex] – [/latex]0.\dot { 4 }[/latex]
∴9x = 4
∴ x = 4/9
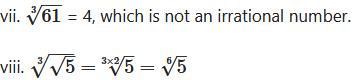
∴ Order = 6
ix. The conjugate of 2√5 + √3 is 2√5 – √3 or -2√5 + √3
x. |12 – (13+7) x 4| = |12 – 20 x 4|
= |12 – 80|
= |-68|
= 68
Solution: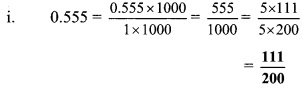

∴ 999x = 29539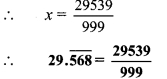

∴ 999x = 9306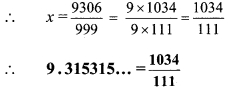
![]()
Since, three numbers i.e. 4, 1 and 7 are repeating after the decimal point.
Thus, multiplying both sides by 1000,
1000x = 357417.417417…
∴ 1000x = 357417.417 …(ii)
Subtracting (i) from (ii),![]()
∴ 999x = 357060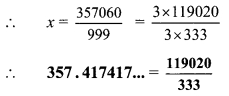
![]()
∴ x = 30.219219
Since, three numbers i.e. 2, 1 and 9 are repeating after the decimal point.
Thus, multiplying both sides by 1000,
1000x= 30219.219219…
∴ 999x = 30189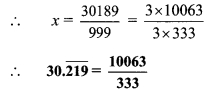
Question 3.
Write the following numbers in its decimal form.
Solution:![]()

ii. 9/11
iii. √5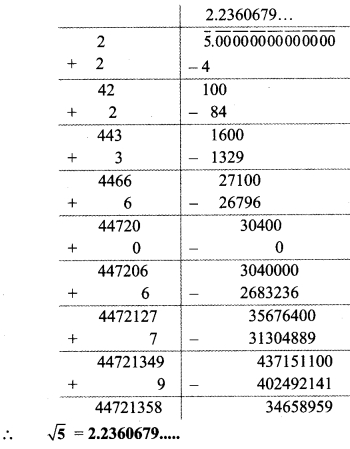

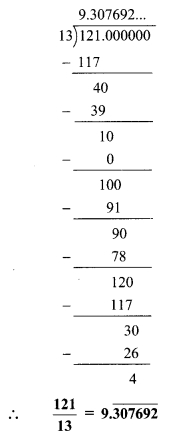
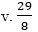

Question 4.
Show that 5 + √7 is an irrational number. [3 Marks]
Solution:
Let us assume that 5 + √7 is a rational number. So, we can find co-prime integers ‘a’ and ‘b’ (b ≠ 0) such that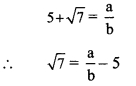
![]()
Our assumption that 5 + √7 is a rational number is wrong.
∴ 5 + √7 is an irrational number.
Question 5.
Write the following surds in simplest form.
Solution:
Question 6.
Write the simplest form of rationalising factor for the given surds.
Solution:![]()
Now, 4√2 x √2 = 4 x 2 = 8, which is a rational number.
∴ √2 is the simplest form of the rationalising factor of √32 .![]()
Now, 5√2 x √2 = 5 x 2 = 10, which is a rational number.
∴ √2 is the simplest form of the rationalising factor of √50 .![]()
Now, 3√3 x √3 = 3 x 3 = 9, which is a rational number.
∴ √ 3 is the simplest form of the rationalising factor of √27 .
= 6, which is a rational number.![]()
![]()
Now, 18√2 x √2 = 18 x 2 = 36, which is a rational number.
∴ √2 is the simplest form of the rationalising factor of 3√72.
vi. 4√11
4√11 x √11 = 4 x 11 = 44, which is a rational number.
∴ √11 is the simplest form of the rationalising factor of 4√11.
Question 7.
Simplify.
Solution:

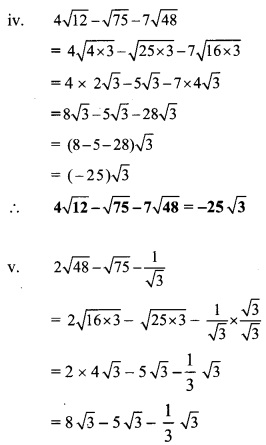
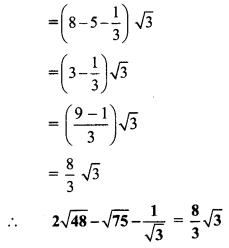
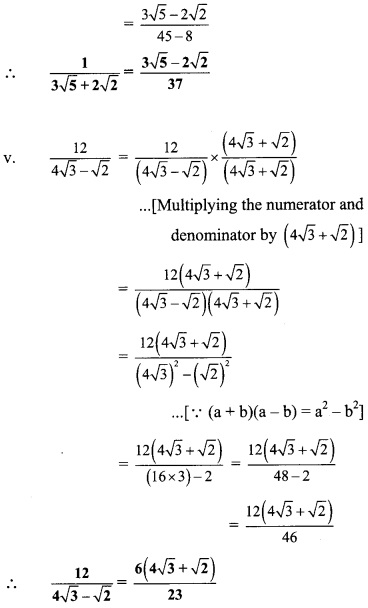
Question 8.
Rationalize the denominator.
Solution: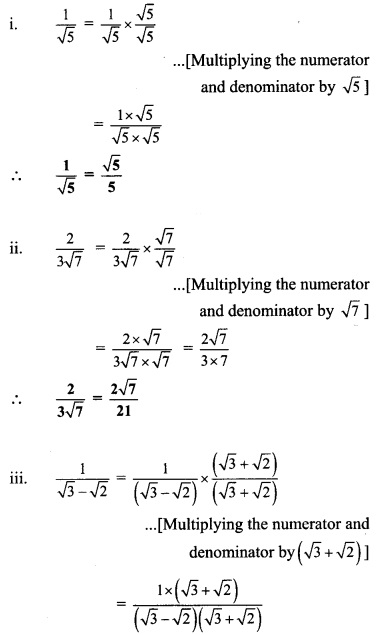

Question 1.
Draw three or four circles of different radii on a card board. Cut these circles. Take a thread and measure the length of circumference and diameter of each of the circles. Note down the readings in the given table. (Textbook pg.no.23 )
Solution:
i. 14,44,3.1
ii. 16,50.3,3.1
iii. 11,34.6,3.1![]()
Question 2.
To find the approximate value of π, take the wire of length 11 cm, 22 cm and 33 cm each. Make a circle from the wire. Measure the diameter and complete the following table.
