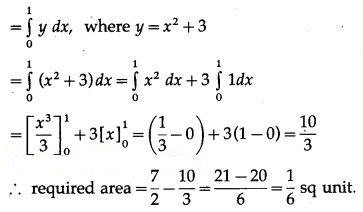1. Find the area of the region bounded by the following curves, X-axis, and the given lines:
(i) y = 2x, x = 0, x = 5.
Solution:

(v) xy = 2, x = 1, x = 4.
Solution: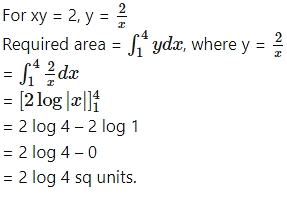
(vi) = x, x = 0, x = 4.
Solution: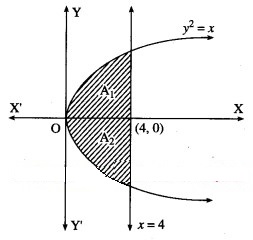
The required area consists of two bounded regions A1 and A2 which are equal in areas.
For = x, y = √x
Required area = 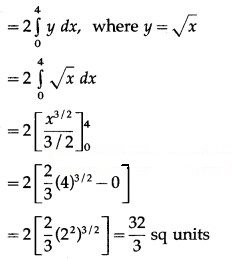
(vii) = 16x, x = 0, x = 4.
Solution: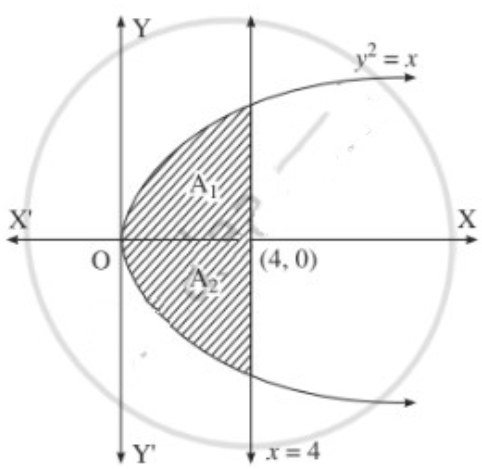
The required area consists of two bounded regions A1 and A2 which are equal in areas.
For = x, y = √x
Required area = 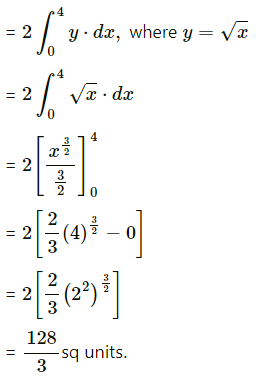
2. Find the area of the region bounded by the parabola:
(i) = 16x and its latus rectum.
Solution:
Comparing = 16x with = 4ax, we get
4a = 16
∴ a = 4
∴ focus is S(a, 0) = (4, 0)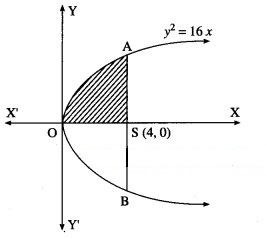
For = 16x, y = 4√x
Required area = area of the region OBSAO
= 2 [area of the region OSAO]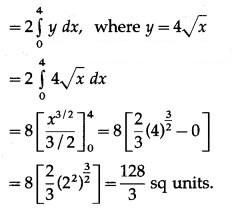
(ii) y = 4 – and the X-axis.
Solution:
The equation of the parabola is y = 4 –
∴ = 4 – y
i.e. (x – 0 = -(y – 4)
It has vertex at P(0, 4)
For points of intersection of the parabola with X-axis,
we put y = 0 in its equation.
∴ 0 = 4 –
∴ = 4
∴ x = ± 2
∴ the parabola intersect the X-axis at A(-2, 0) and B(2, 0)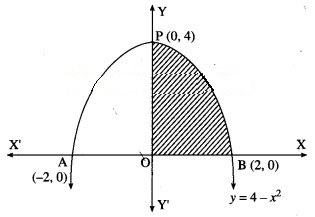
Required area = area of the region APBOA
= 2[area of the region OPBO]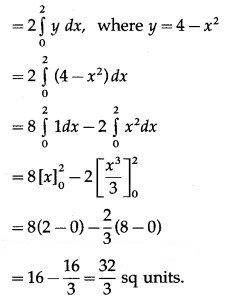
3. Find the area of the region included between:
(i) = 2x and y = 2x.
Solution:
The vertex of the parabola = 2x is at the origin O = (0, 0).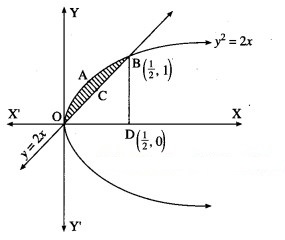
To find the points of intersection of the line and the parabola, equaling the values of 2x from both the equations we get,
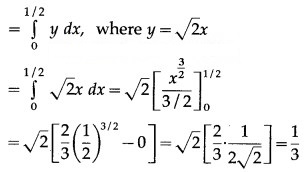
Area of the region OCBDO = area under the line y = 2x between x = 0 and x = 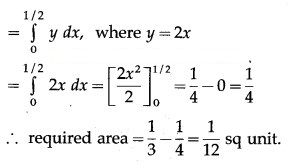
(ii) = 4x and y = x.
Solution:
The vertex of the parabola = 4x is at the origin O = (0, 0).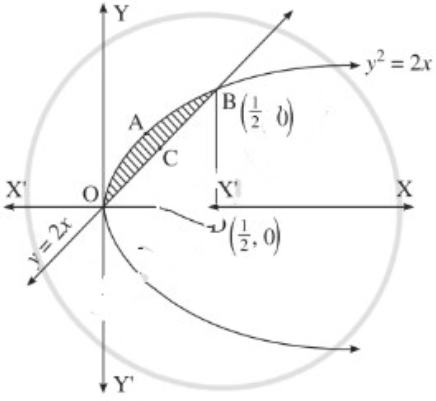
To find the points of intersection of the line and the parabola, equaling the values of 4x from both the equations we get,
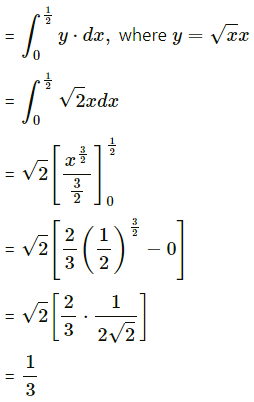
Area of the region OCBDO = area under the line y = 2x between x = 0 and x = 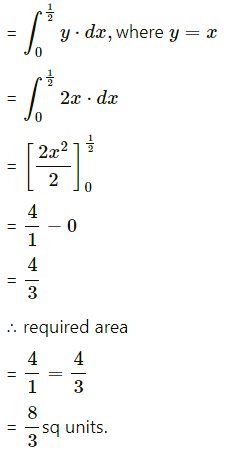
(iii) y = and the line y = 4x.
Solution:
The vertex of the parabola y = is at the origin 0(0, 0)
To find the points of the intersection of a line and the parabola.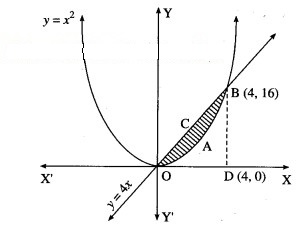
Equating the values of y from the two equations, we get
= 4x
∴ – 4x = 0
∴ x(x – 4) = 0
∴ x = 0, x = 4
When x = 0, y = 4(0) = 0
When x = 4, y = 4(4) = 16
∴ the points of intersection are 0(0, 0) and B(4, 16)
Required area = area of the region OABCO = (area of the region ODBCO) – (area of the region ODBAO)
Now, area of the region ODBCO = area under the line y = 4x between x = 0 and x = 4
(iv) = 4ax and y = x.
Solution:
The vertex of the parabola = 4ax is at the origin O = (0, 0).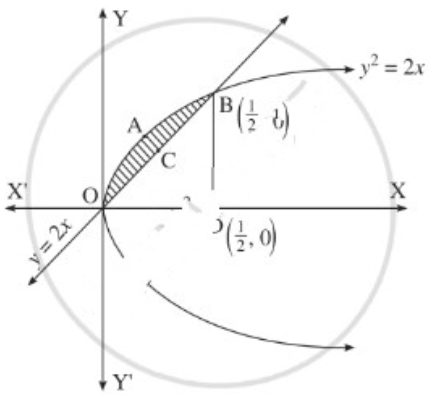
To find the points of intersection of the line and the parabola, equaling the values of 4ax from both the equations we get,
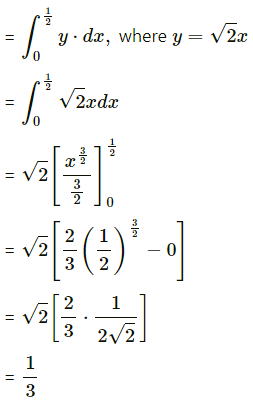
Area of the region OCBDO
= area under the line y![]()
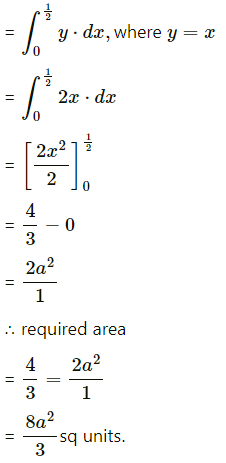
(v) y = + 3 and y = x + 3.
Solution:
The given parabola is y = + 3, i.e. (x – 0 = y – 3
∴ its vertex is P(0, 3).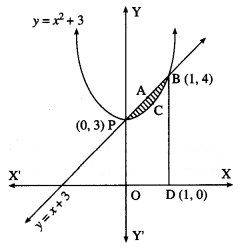
To find the points of intersection of the line and the parabola.
Equating the values of y from both the equations, we get
+ 3 = x + 3
∴ – x = 0
∴ x(x – 1) = 0
∴ x = 0 or x = 1
When x = 0, y = 0 + 3 = 3
When x = 1, y = 1 + 3 = 4
∴ the points of intersection are P(0, 3) and B(1, 4)
Required area = area of the region PABCP = area of the region OPABDO – area of the region OPCBDO
Now, area of the region OPABDO
= area under the line y = x + 3 between x = 0 and x = 1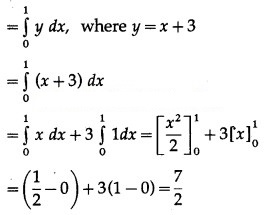
Area of the region OPCBDO = area under the parabola y = + 3 between x = 0 and x = 1