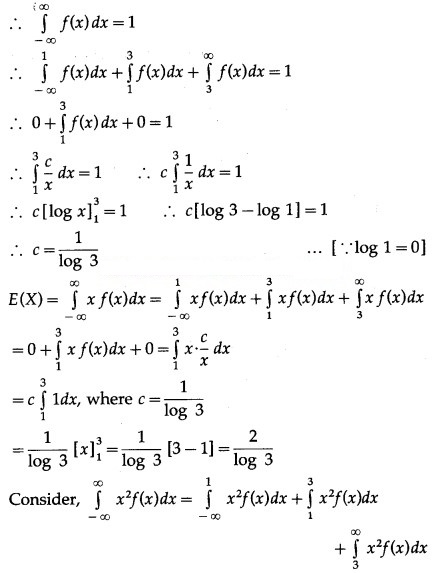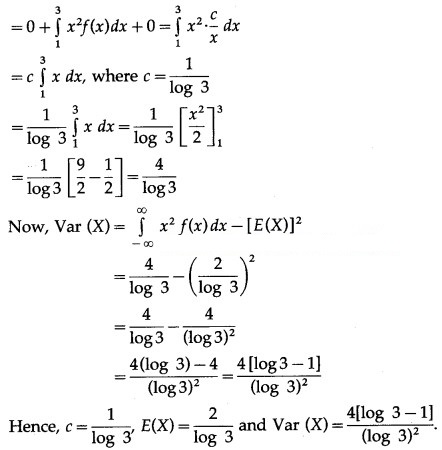Question 1.
Verify which of the following is p.d.f. of r.v. X:![]()
(ii) f(x) = x, for 0 ≤ x ≤ 1 and -2 – x for 1 < x < 2
(iii) fix) = 2, for 0 ≤ x ≤ 1.
Solution:
f(x) is the p.d.f. of r.v. X if
(a) f(x) ≥ 0 for all x ∈ R and
Hence, f(x) is the p.d.f. of X.
(ii) f(x) = x ≥ 0 if 0 ≤ x ≤ 1
For 1 < x < 2, -2 < -x < -1
-2 – 2 < -2 – x < -2 – 1
i.e. -4 < f(x) < -3 if 1 < x < 2
Hence, f(x) is not p.d.f. of X.
(iii) (a) f(x) = 2 ≥ 0 for 0 ≤ x ≤ 1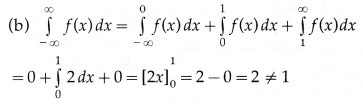
Hence, f(x) is not p.d.f. of X.
Question 2.
The following is the p.d.f. of r.v. X:
f(x) = x/8, for 0 < x < 4 and = 0 otherwise.
Find
(a) P(x < 1.5)
(b) P(1 < x < 2) (c) P(x > 2).
Solution: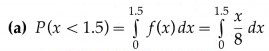
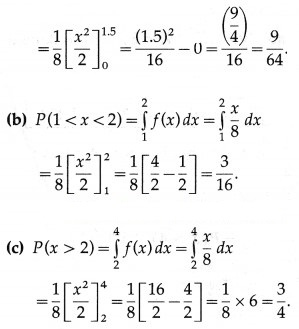
Question 3.
It is known that error in measurement of reaction temperature (in 0°C) in a certain experiment is continuous r.v. given by![]()
= 0. otherwise.
(i) Verify whether f(x) is p.d.f. of r.v. X
(ii) Find P(0 < x ≤ 1)
(iii) Find the probability that X is negative.
Solution:
Question 4.
Solution:
(i) Since, the function f is p.d.f. of X
(ii) Since, the function f is the p.d.f. of X,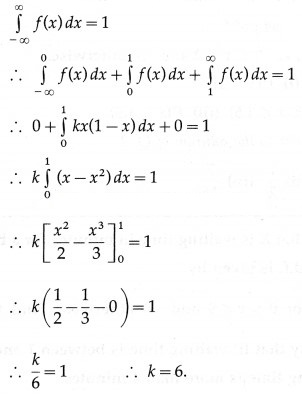

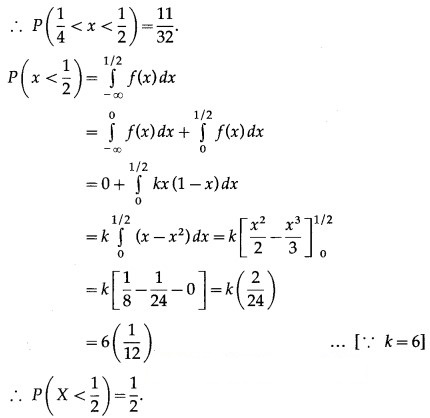
Question 5.
Let X be the amount of time for which a book is taken out of the library by a randomly selected students and suppose X has p.d.f.
f(x) = 0.5x, for 0 ≤ x ≤ 2 and = 0 otherwise.
Calculate:
(i) P(x ≤ 1)
(ii) P(0.5 ≤ x ≤ 1.5)
(iii) P(x ≥ 1.5).
Solution:
(i) P(x ≤ 1)
(ii) P(0.5 ≤ x ≤ 1.5)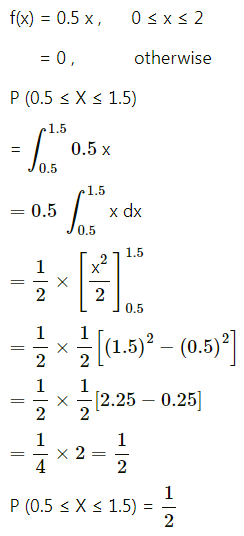
(iii) P(x ≥ 1.5)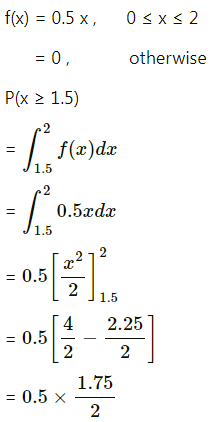
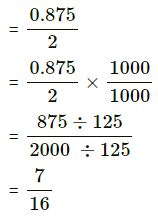
Question 6.
Suppose that X is waiting time in minutes for a bus and its p.d.f. is given by f(x) = 1/5, for 0 ≤ x ≤ 5 and = 0 otherwise. Find the probability that
(i) waiting time is between 1 and 3
(ii) waiting time is more than 4 minutes.
Solution:
(i) Required probability = P(1 < X < 3)
(ii) Required probability = P(X > 4)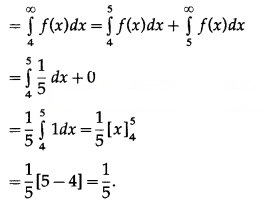
Question 7.
Suppose the error involved in making a certain measurement is a continuous r.v. X with p.d.f.
f(x) = k(4 – ), -2 ≤ x ≤ 2 and 0 otherwise.
Compute:
(i) P(X > 0)
(ii) P(-1 < X < 1)
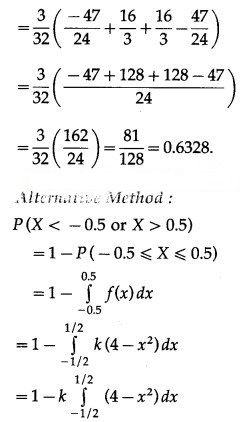
(iii) P(-0.5 < X or X > 0.5).
Solution:
Since, f is the p.d.f. of X,
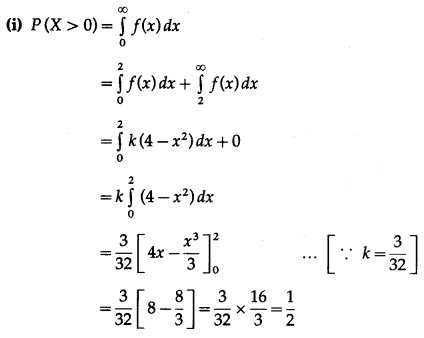
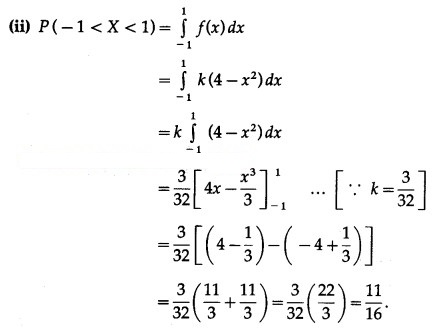
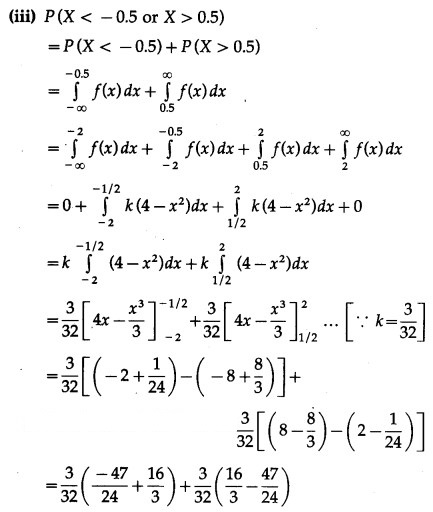

Question 8.
The following is the p.d.f. of continuous r.v. X
f(x) = x/8, for 0 < x < 4 and = 0 otherwise.
(i) Find expression for c.d.f. of X.
(ii) Find F(x) at x = 0.5, 1.7 and 5.
Solution:
(i) Let F(x) be the c.d.f. of X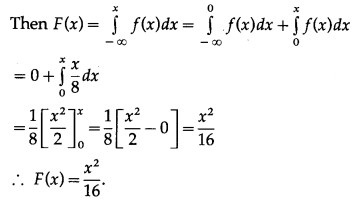

Question 9.
Given the p.d.f. of a continuous random r.v. X, f(x) = , for -1 < x < 2 and = 0 otherwise. Determine c.d.f. of X and hence find P(X < 1); P(X < -2), P(X > 0), P(1 < X < 2).
Solution: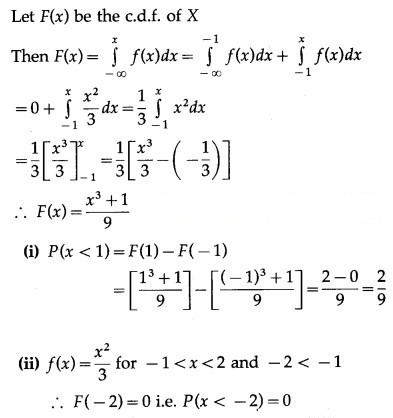

Question 10.
If a r.v. X has p.d.f.
f(x) = c/x for 1 < x < 3, c > 0. Find c, E(X), Var (X).
Solution:
Since f(x) is p.d.f of r.v. X