Question 1.
For the following G.P.s, find .
(i) 3, 6, 12, 24, ……..
Solution: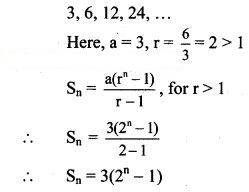
Solution: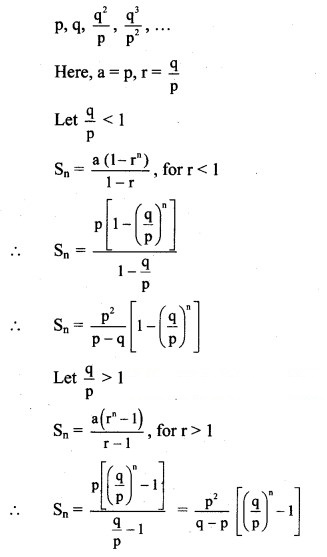
(iii) 0.7, 0.07, 0.007, …….
Solution: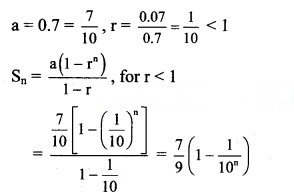
(iv) √5, -5, 5√5, -25, …….
Solution: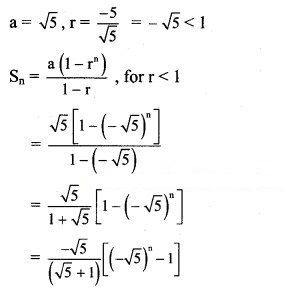
Question 2.
For a G.P.
Solution: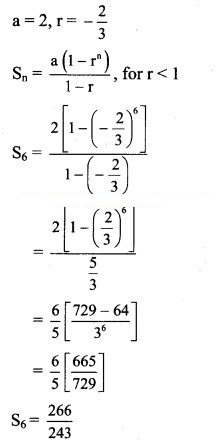
(ii) If = 1023, r = 4, find a.
Solution: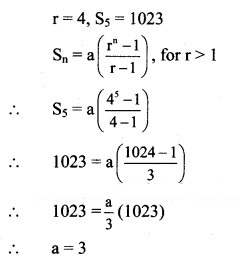
Question 3.
For a G.P.
(i) If a = 2, r = 3, = 242, find n.
Solution: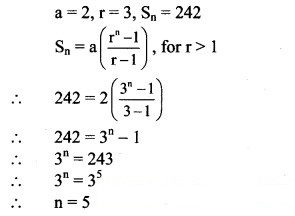
(ii) For a G.P. sum of the first 3 terms is 125 and the sum of the next 3 terms is 27, find the value of r.
Solution:
Question 4.
For a G.P.
Solution:
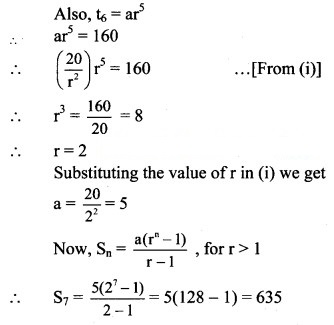
Solution:
Question 5.
Find the sum to n terms
(i) 3 + 33 + 333 + 3333 + …..
Solution: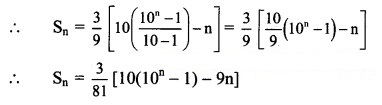
(ii) 8 + 88 + 888 + 8888 + …..
Solution:

Question 6.
Find the sum to n terms
(i) 0.4 + 0.44 + 0.444 + …..
Solution: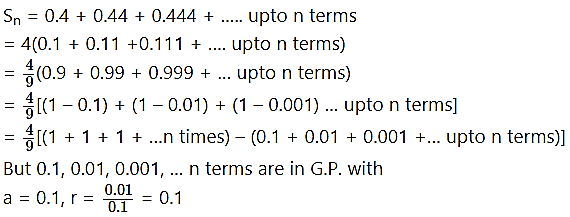
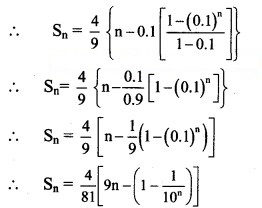
(ii) 0.7 + 0.77 + 0.777 + ……
Solution:
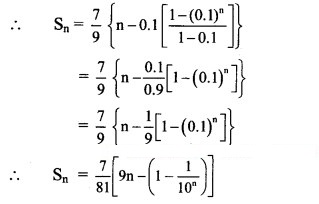
Question 7.
Find the sum to n terms of the sequence
(i) 0.5, 0.05, 0.005, …..
Solution:
(ii) 0.2, 0.02, 0.002, ……
Solution: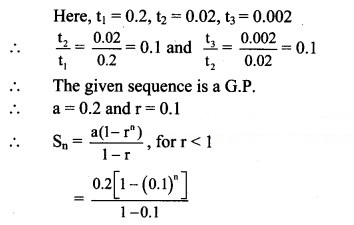

Question 8.
For a sequence, if = 2(3n – 1), find the nth term, hence showing that the sequence is a G.P.
Solution:
Question 9.
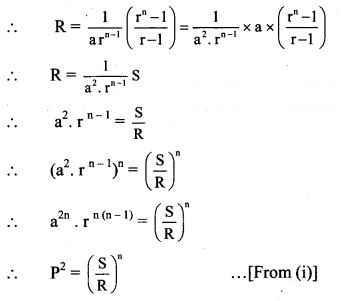
Question 10.
Solution:
Let a and r be the 1st term and common ratio of the G.P. respectively.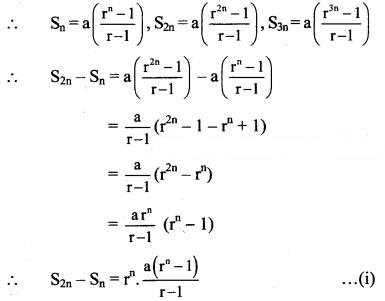
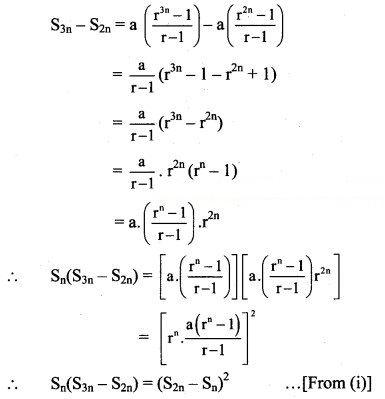
Question 11.
Find
Solution:
Solution: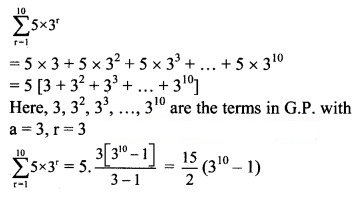
Question 12.
The value of a house appreciates 5% per year. How much is the house worth after 6 years if its current worth is Rs. 15 Lac. [Given: (1.05 = 1.28, (1.05 = 1.34]
Solution:
The value of a house is Rs. 15 Lac.
Appreciation rate = 5% = 5/100 = 0.05
Value of house after 1st year = 15(1 + 0.05) = 15(1.05)
Value of house after 6 years = 15(1.05) (1.05
= 15(1.05
= 15(1.34)
= 20.1 lac.
Question 13.
If one invests Rs. 10,000 in a bank at a rate of interest 8% per annum, how long does it take to double the money by compound interest? [(1.08 = 1.47]
Solution:
Amount invested = Rs. 10000
Interest rate = 8/100 = 0.08
amount after 1st year = 10000(1 + 0.08) = 10000(1.08)
Value of the amount after n years
= 10000(1.08) × (1.08
= 10000(1.08
= 20000
∴ (1.08 = 2
∴ (1.08 = 1.47 …..[Given]
∴ n = 10 years, (approximately)
