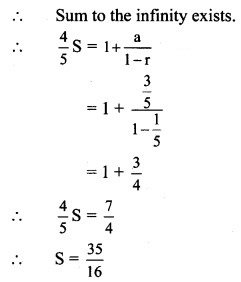(I) Select the correct answer from the given alternative:
Question 1.
The common ratio for the G.P. 0.12, 0.24, 0.48, is
(A) 0.12
(B) 0.2
(C) 0.02
(D) 2
Answer:
(D) 2
Question 2.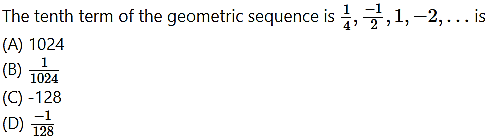
Answer:
(C) -128
Hint: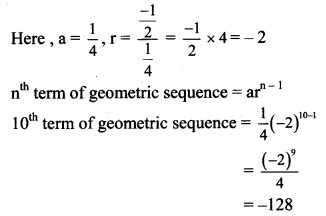
Question 3.
(A) 3
(B) 2
(C) 1
(D) -1
Answer:
(A) 3
Hint: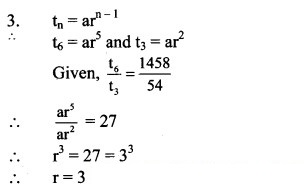
Question 4.
Which term of the geometric progression 1, 2, 4, 8, ….. is 2048.
(A) 10th
(B) 11th
(C) 12th
(D) 13th
Answer:
(C) 12th
Hint:
Here, a = 1, r = 2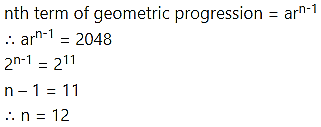
Question 5.
If the common ratio of the G.P. is 5, the 5th term is 1875, the first term is
(A) 3
(B) 5
(C) 15
(D) -5
Answer:
(A) 3
Question 6.
The sum of 3 terms of a G.P. is 21/4 and their product is 1, then the common ratio is
(A) 1
(B) 2
(C) 4
(D) 8
Answer:
(C) 4
Hint:
Question 7.

Question 9.
Which of the following is not true, where A, G, H are the AM, GM, HM of a and b respectively, (a, b > 0)
Answer:
(D) A = GH
Question 10.
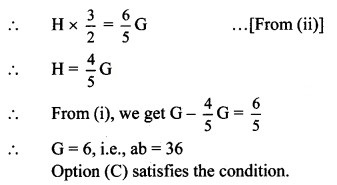
The G.M. of two numbers exceeds their H.M. by 6/5, the A.M. exceeds G.M. by 3/2 the two numbers are
Answer:
(C) 3, 12
Hint:
(II) Answer the following:
Question 1.
In a G.P., the fourth term is 48 and the eighth term is 768. Find the tenth term.
Solution:
Question 2.
Find the sum of the first 5 terms of the G.P. whose first term is 1 and the common ratio is 2/3.
Solution: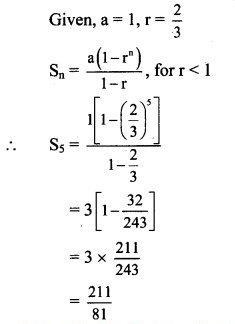
Question 3.
Solution: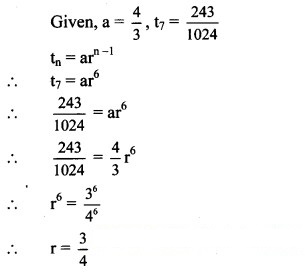
Question 4.
Question 5.
Find three numbers in G.P. such that their sum is 35 and their product is 1000.
Solution:
Substituting the value of a in (i), we get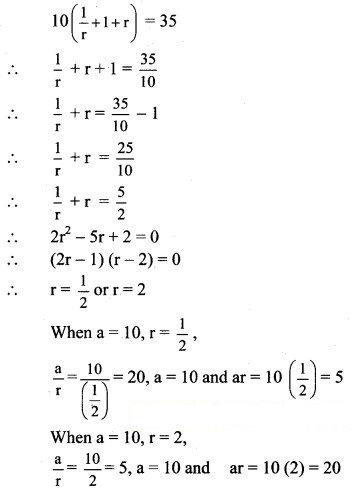
Hence, the three numbers in G.P. are 20, 10, 5, or 5, 10, 20.
Question 6.
Find five numbers in G.P. such that their product is 243 and the sum of the second and fourth numbers is 10.
Solution:![]()
According to the given condition,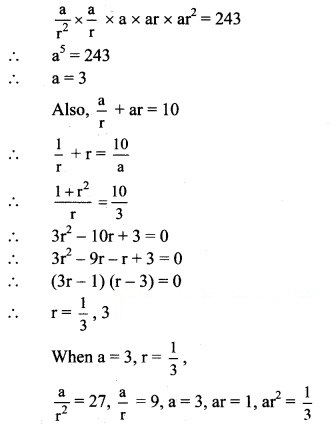
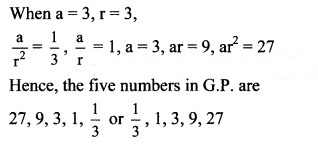
Question 7.
For a sequence, Sn = 4( – 1), verify that the sequence is a G.P.
Solution: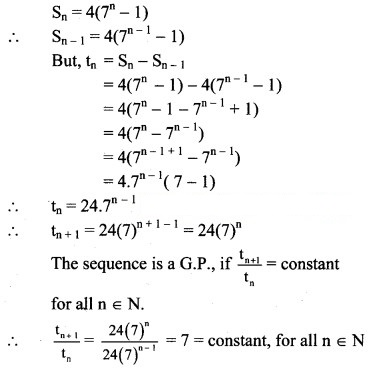
∴ The given sequence is a G.P.
Question 8.
Find 2 + 22 + 222 + 2222 + … upto n terms.
Solution: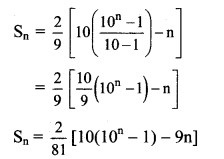
Question 9.
Find the nth term of the sequence 0.6, 0.66, 0.666, 0.6666,…
Solution:
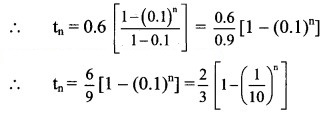
Question 10.
Solution: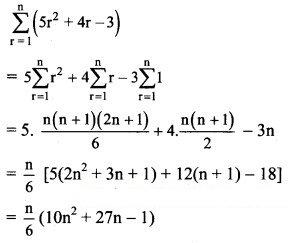
Question 11.
Solution: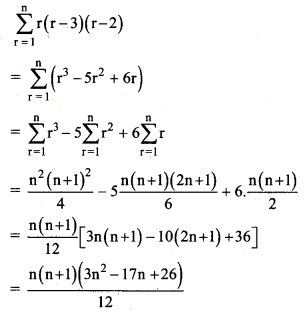
Question 12.
Solution:
We know that
Question 13.
Solution: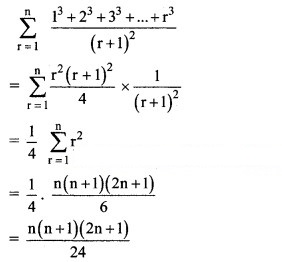
Question 14.
Find 2 × 6 + 4 × 9 + 6 × 12 + ….. upto n terms.
Solution:
2, 4, 6, ….. are in A.P.
∴ rth term = 2 + (r – 1) 2 = 2r
6, 9, 12, ….. are in A.P.
∴ rth term = 6 + (r – 1)(3) = (3r + 3)
∴ 2 × 6 + 4 × 9 + 6 × 12 + ….. to n terms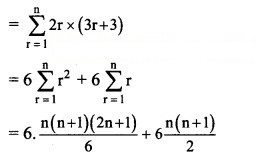
= n(n + 1) [2n + 1 + 3]
= 2n(n + 1)(n + 2)
Question 15.
Find 2 × 5 × 8 + 4 × 7 × 10 + 6 × 9 × 12 + …… upto n terms.
Solution:
2, 4, 6,… are in A.P.
∴ rth term = 2 + (r – 1) 2 = 2r
5, 7, 9, … are in A.P.
∴ rth term = 5 + (r – 1) (2) = (2r + 3)
8, 10, 12, … are in A.P.
∴ rth term = 8 + (r – 1) (2) = (2r + 6)
2 × 5 × 8 + 4 × 7 × 10 + 6 × 9 × 12 + ….. to n terms
= 2n (n + 1) [n(n + 1) + 3(2n + 1) + 9]
= 2n (n + 1)( + 7n + 12)
= 2n (n + 1) (n + 3) (n + 4)
Question 16.
Solution: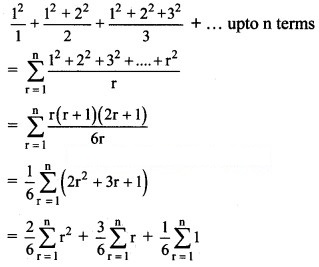
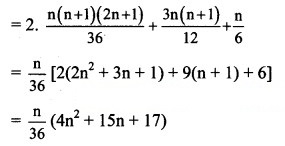
Question 17.
Solution: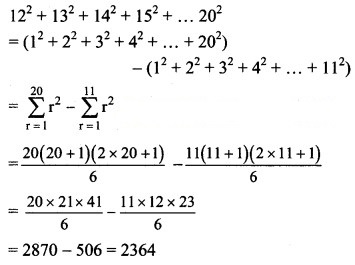
Question 18.
Solution: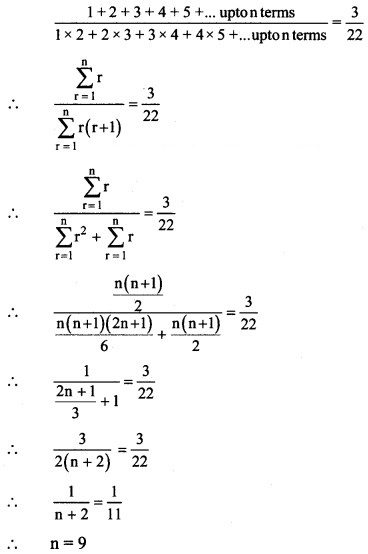
Question 19.
Solution: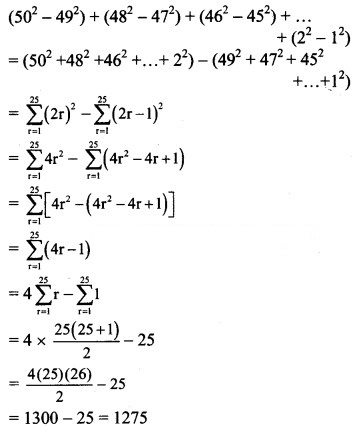
Question 20.
Solution:
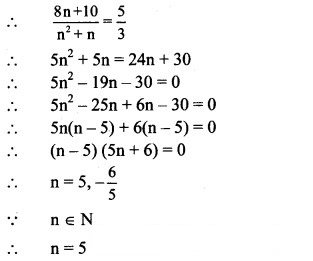
Question 21.
For a G.P. if = 7, = 1575, find a.
Solution: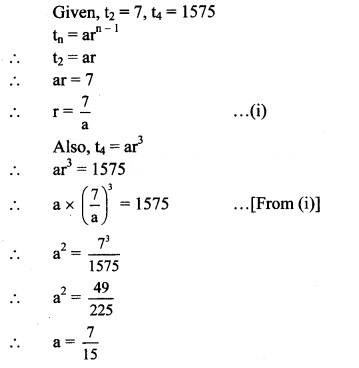
Question 22.
Solution: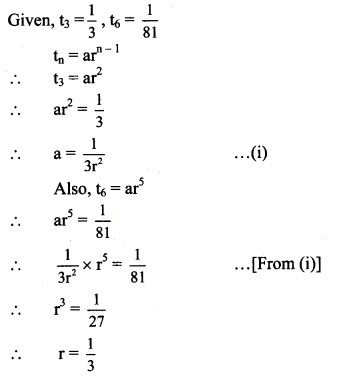
Question 23.
Solution: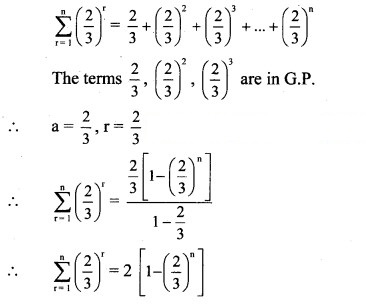
Question 24.
Find k so that k – 1, k, k + 2 are consecutive terms of a G.P.
Solution:
Since k – 1, k, k + 2 are consecutive terms of a G.P.,
k – 2 = 0
∴ k = 2
Question 25.
If for a G.P. first term is (27 and the seventh term is (8, find .
Solution: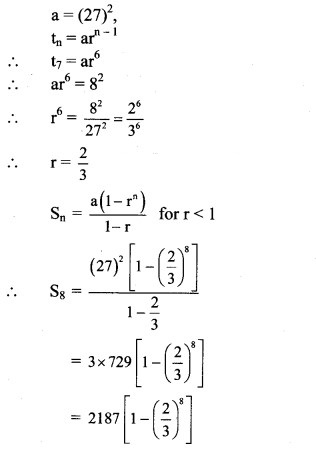
Question 26.
If pth, qth and rth terms of a G.P. are x, y, z respectively. Find the value of .
Solution:
Let a be the first term and R be the common ratio of the G.P.
Question 27.
Which 2 terms are inserted between 5 and 40 so that the resulting sequence is G.P.
Solution:
Let the required numbers be G1 and G2.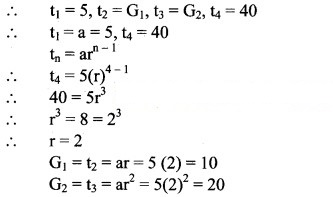
∴ For the resulting sequence to be in G.P. we need to insert numbers 10 and 20.
Question 28.
Solution:
Question 29.
Solution:
a, b, c are in G.P.
∴ = ac
a + 2bx + c = 0 becomes
Question 30.
Solution:
p, q, r, s are in G.P.
Question 31.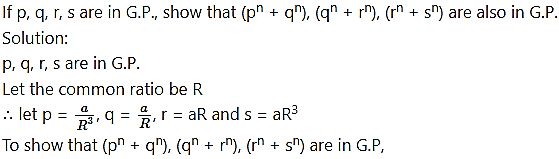
i.e., we have to show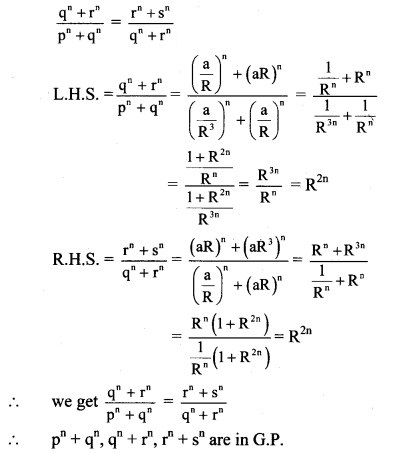
Question 32.
Find the coefficient in the expression of using series expansion.
Solution: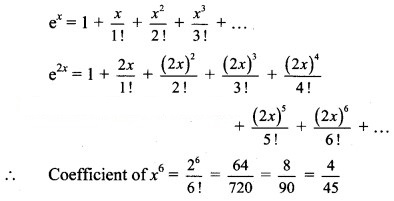
Question 33.
Solution: