Question 1.
Examine the continuity of
(i) f(x) = x3 + 2 x2 – x – 2 at x = -2
Solution:
Given, f(x) = x3 + 2 x2 – x – 2
f(x) is a polynomial function and hence it is continuous for all x ∈ R.
∴ f(x) is continuous at x = -2.
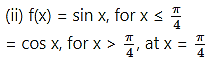
Solution:
= 8 for x = 3, at x = 3.
Solution:
f(3) = 8 ….(given)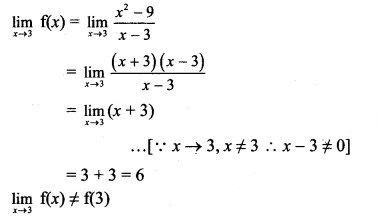
∴ f(x) is discontinuous at x = 3.
Question 2.
Examine whether the function is continuous at the points indicated against them.
(i) f(x) = x2 – 2x + 1, if x ≤ 2
= 3x – 2, if x > 2, at x = 2.
Solution: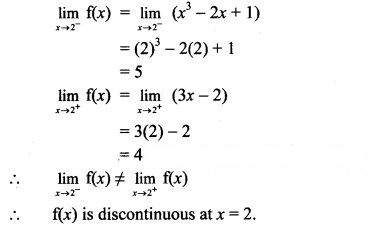
= 20, for x = 1, at x = 1.
Solution: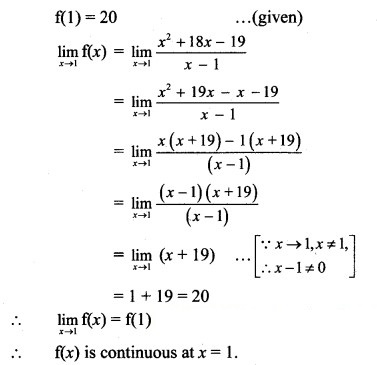
Solution: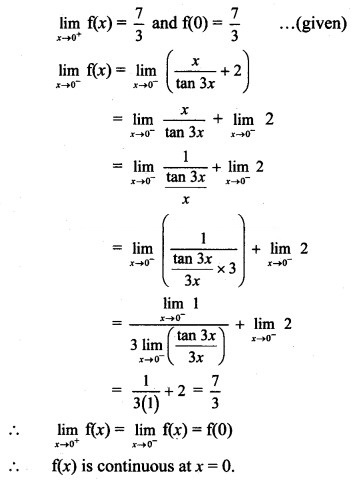
Question 3.
Find all the points of discontinuities of f(x) = [x] on the interval (-3, 2).
Solution:
f(x) = [x], x ∈ (-3, 2)
i.e., f(x) = -3, x ∈ (-3, -2)
= -2, x ∈ [-2, -1)
= -1, x ∈ [- 1, 0)
= 0, x ∈ [0, 1)
= 1, x ∈ [1, 2)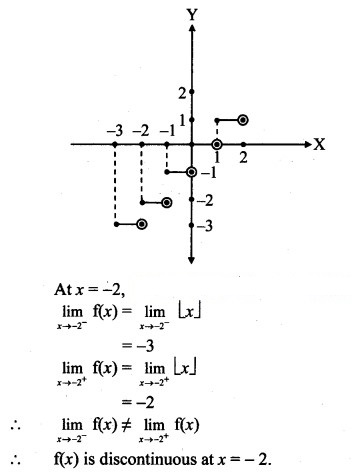
Similarly, f(x) is discontinuous at the points x = -1, x = 0, x = 1.
Thus all the integer values of x in the interval (-3, 2),
i.e., the points x = -2, x = -1, x = 0 and x = 1 are the required points of discontinuities.
Question 4.
Solution: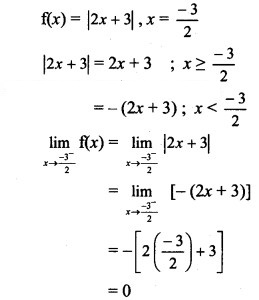
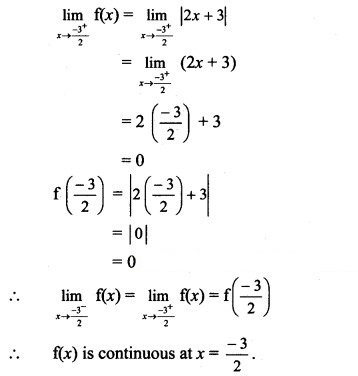
Question 5.
Test the continuity of the following functions at the points or intervals indicated against them.
Solution: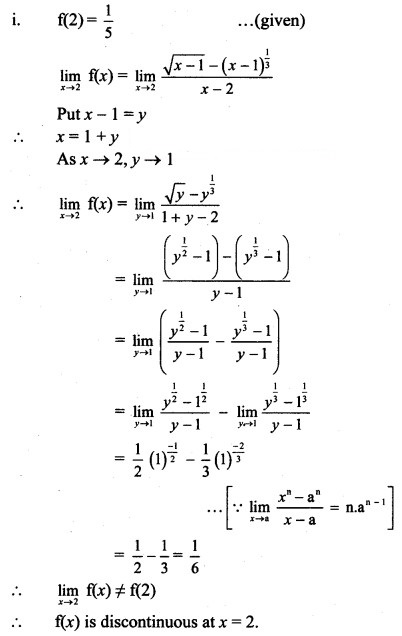
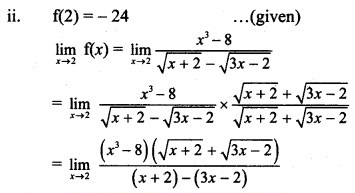
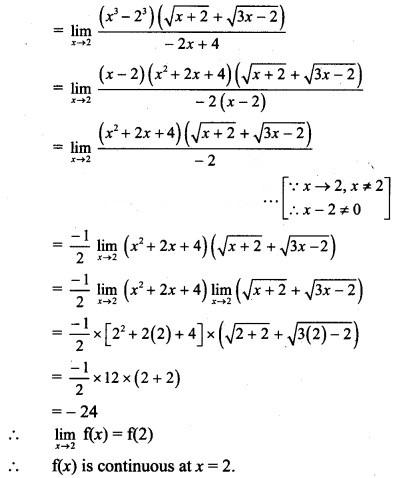
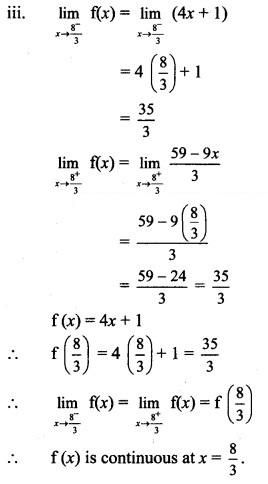
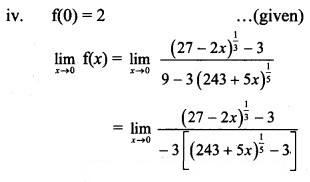

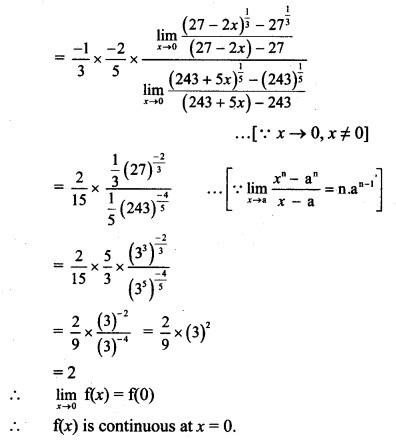
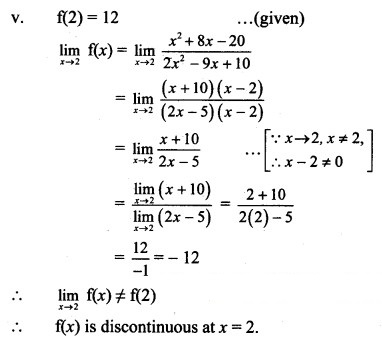
Question 6.
Identify discontinuities for the following functions as either a jump or a removable discontinuity.
It is a rational function and is discontinuous if
x – 7 = 0, i.e., x = 7
∴ f(x) is continuous for all x ∈ R, except at x = 7.
∴ f(7) is not defined.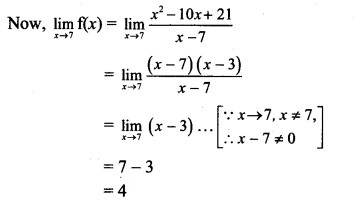
Thus, limx→7f(x) exist but f(7) is not defined.
∴ f(x) has a removable discontinuity.
(ii) f(x) = x2 + 3x – 2, for x ≤ 4
= 5x + 3, for x > 4.
Solution:
f(x) = x2 + 3x – 2, x ≤ 4
= 5x + 3, x > 4
f(x) is a polynomial function for both the intervals.
∴ f(x) is continuous for both the given intervals.
Let us test the continuity at x = 4.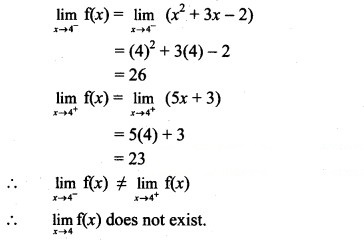
∴ f(x) is discontinuous at x = 4.
∴ f(x) has a jump discontinuity at x = 4.
(iii) f(x) = x2 – 3x – 2, for x < -3 = 3 + 8x, for x > -3.
Solution:
f(x) = x2 – 3x – 2, x < -3 = 3 + 8x, x > -3
f(x) is a polynomial function for both the intervals.
∴ f(x) is continuous for both the given intervals.
Let us test the continuity at x = -3.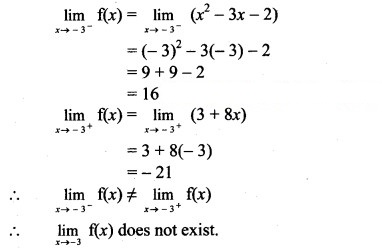
∴ f(x) is discontinuous at x = -3.
∴ f(x) has a jump discontinuity at x = -3
(iv) f(x) = 4 + sin x, for x < π = 3 – cos x for x > π.
Solution:
f(x) = 4 + sin x, x < π = 3 – cos x, x > π
sin x and cos x are continuous for all x ∈ R.
4 and 3 are constant functions.
∴ 4 + sin x and 3 – cos x are continuous for all x ∈ R.
∴ f(x) is continuous for both the given intervals.
Let us test the continuity at x = π.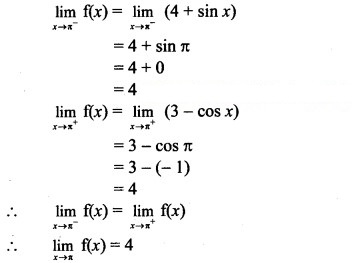
But f(π) is not defined.
∴ f(x) has a removable discontinuity at x = π.
Question 7.
Show that the following functions have a continuous extension to the point where f(x) is not defined. Also, find the extension.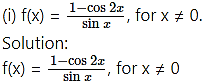
Here, f(0) is not defined.
Consider,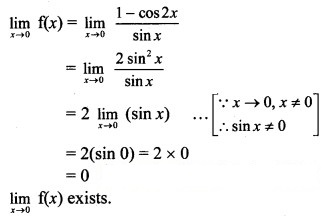
But f(0) is not defined.
∴ f(x) has a removable discontinuity at x = 0.
∴ The extension of the original function is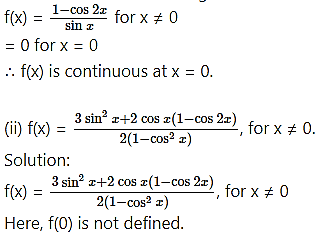
Consider,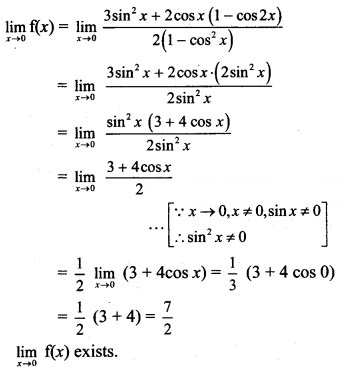
But f(0) is not defined.
∴ f(x) has a removable discontinuity at x = 0.
∴ The extension of the original function is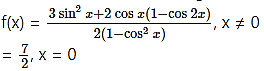
∴ f(x) is continuous at x = 0.
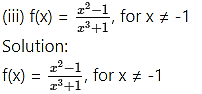
Here, f(-1) is not defined.
Consider,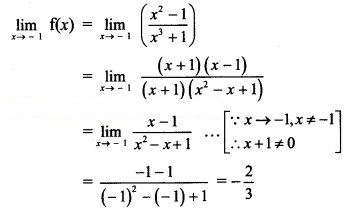
But f(-1) is not defined.
∴ f(x) has a removable discontinuity at x = -1.
∴ The extension of the original function is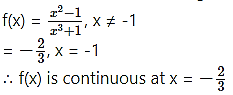
Question 8.
Discuss the continuity of the following functions at the points indicated against them.
Solution: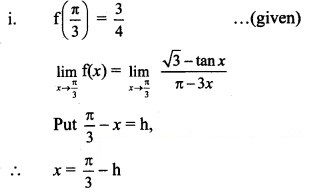

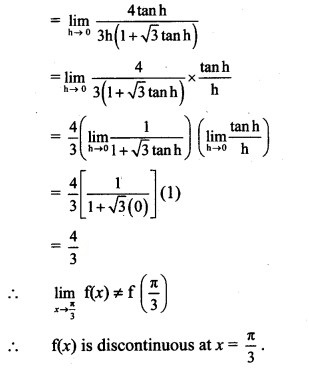
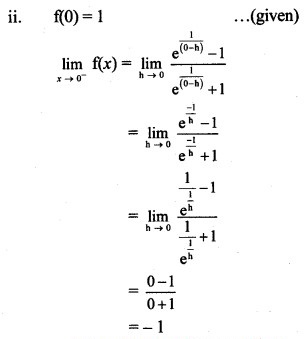
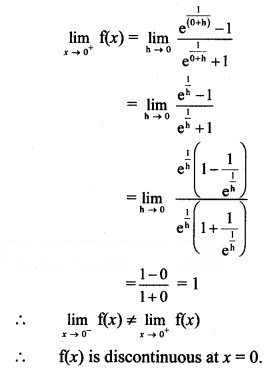

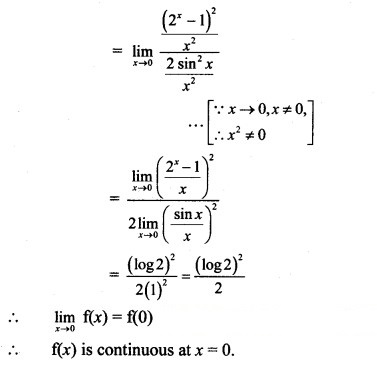
Question 9.
Which of the following functions has a removable discontinuity? If it has a removable discontinuity, redefine the function so that it becomes continuous.
Solution:
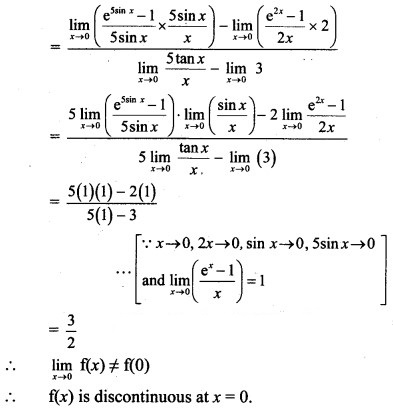

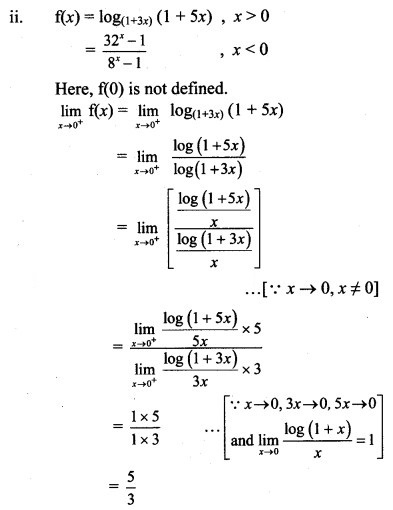
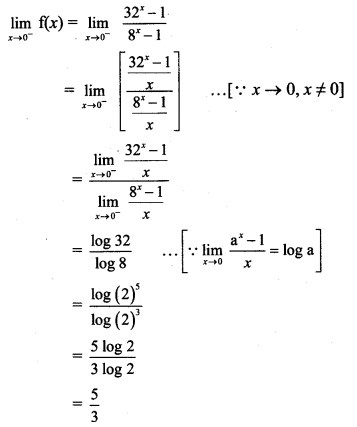

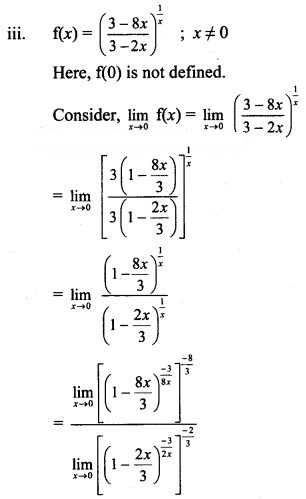


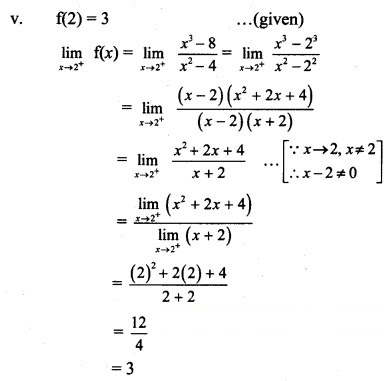
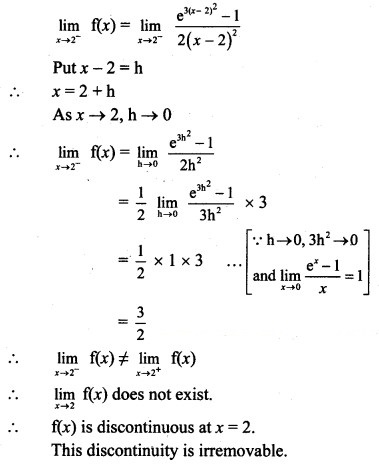
Question 10.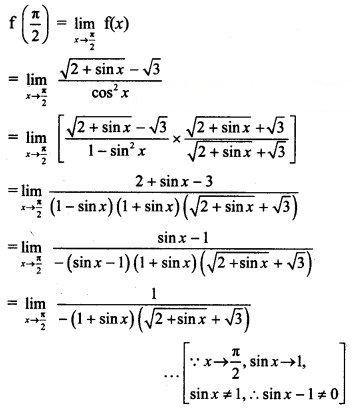
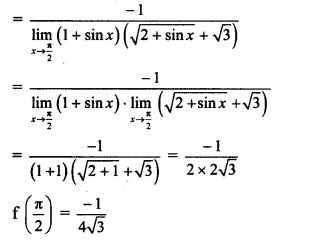
Solution:
f(x) is continuous at x = 0, …..(given)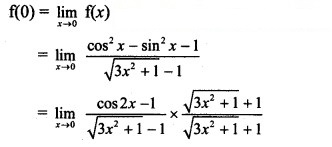
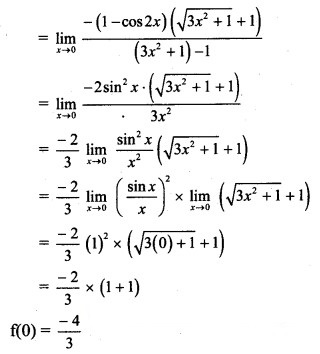
Solution:
f(x) is continuous at x = π, …..(given)
Question 11.
= k, for x = 0
is continuous at x = 0, then find k.
Solution:
f(x) is continuous at x = 0 …..(given)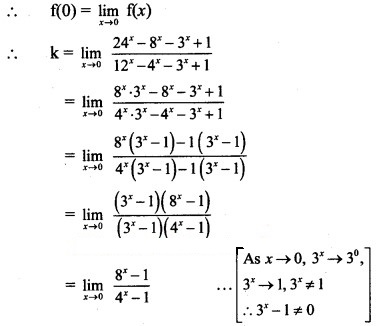
= k, for x = 0
is continuous at x = 0, then find k.
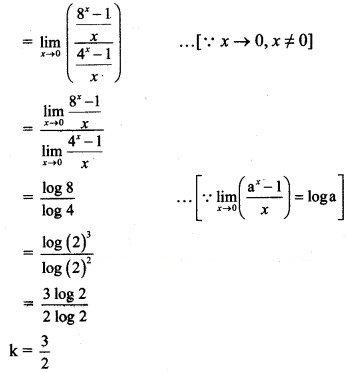
Solution:
f(x) is continuous at x = 0 …..(given)
= 4 for x = 0
= x2 + b – 3, for x < 0
is continuous at x = 0, find a and b.
Solution:
f(x) is continuous at x = 0 ……(given)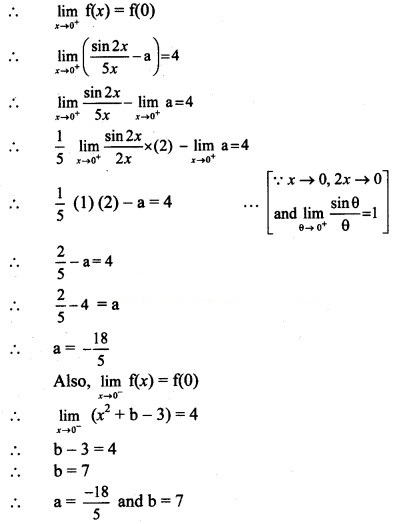
(iv) For what values of a and b is the function
f(x) = ax + 2b + 18, for x ≤ 0
= x2 + 3a – b, for 0 < x ≤ 2 = 8x – 2, for x > 2,
continuous for every x?
Solution:
f(x) is continuous for every x …..(given)
∴ f(x) is continuous at x = 0 and x = 2.
As f(x) is continuous at x = 0,
limx→0−f(x)=limx→0+f(x)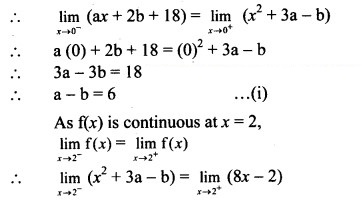
∴ (2)2 + 3a – b = 8(2) – 2
∴ 4 + 3a – b = 14
∴ 3a – b = 10 …….(ii)
Subtracting (i) from (ii), we get
2a = 4
∴ a = 2
Substituting a = 2 in (i), we get
2 – b = 6
∴ b = -4
∴ a = 2 and b = -4
(v) For what values of a and b is the function
= 2x – a + b, for x ≥ 3
continuous for every x on R?
Solution:
f(x) is continuous for every x on R …..(given)
∴ f(x) is continuous at x = 2 and x = 3.
As f(x) is continuous at x = 2,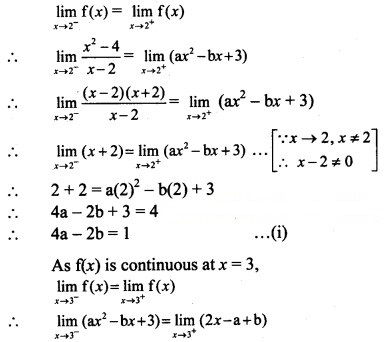
∴ a(3)2 – b(3) + 3 = 2(3) – a + b
∴ 9a – 3b + 3 = 6 – a + b
∴ 10a – 4b = 3 …..(ii)
Multiplying (i) by 2, we get
8a – 4b = 2 ….(iii)
Subtracting (ii) from (iii), we get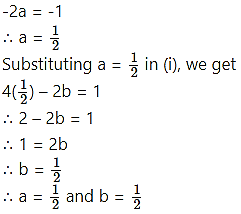
Question 12.
Discuss the continuity of f on its domain, where
f(x) = |x + 1|, for -3 ≤ x ≤ 2
= |x – 5|, for 2 < x ≤ 7
Solution:
Question 13.
Solution: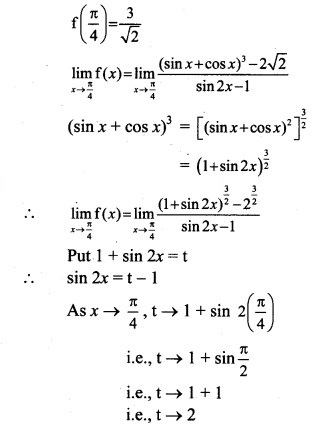
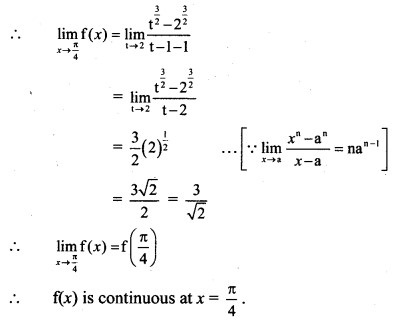
Question 14.
Determine the values of p and q such that the following function is continuous on the entire real number line.
f(x) = x + 1, for 1 < x < 3
= x2 + px + q, for |x – 2| ≥ 1.
Solution:
|x – 2| ≥ 1
∴ x – 2 ≥ 1 or x – 2 ≤ -1
∴ x ≥ 3 or x ≤ 1
∴ f(x) = x2 + px + q for x ≥ 3 as well as x ≤ 1
Thus, f(x) = x2 + px + q; x ≤ 1
= x + 1; 1 < x < 3 = x2 + px + q; x > 3
f(x) is continuous for all x ∈ R.
∴ f(x) is continuous at x = 1 and x = 3.
As f(x) is continuous at x = 1,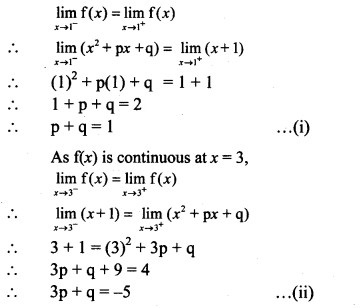
Subtracting (i) from (ii), we get
2p = -6
∴ p = -3
Substituting p = -3 in (i), we get
-3 + q = 1
∴ q = 4
∴ p = -3 and q = 4
Question 15.
Show that there is a root for the equation 2x3 – x – 16 = 0 between 2 and 3.
Solution:
Let f(x) = 2x3 – x – 16
f(x) is a polynomial function and hence it is continuous for all x ∈ R.
A root of f(x) exists, if f(x) = 0 for at least one value of x.
f(2) = 2(2)3 – 2 – 16 = -2 < 0
f(3) = 2(3)3 – 3 – 16 = 35 > 0
∴ f(2) < 0 and f(3) > 0
∴ By intermediate value theorem,
there has to be point ‘c’ between 2 and 3 such that f(c) = 0.
∴ There is a root of the given equation between 2 and 3.
Question 16.
Show that there is a root for the equation x3 – 3x = 0 between 1 and 2.
Solution:
Let f(x) = x3 – 3x
f(x) is a polynomial function and hence it is continuous for all x ∈ R.
A root of f(x) exists, if f(x) = 0 for at least one value of x.
f(1) = (1)3 – 3(1) = -2 < 0
f(2) = (2)3 – 3(2) = 2 > 0
∴ f(1) < 0 and f(2) > 0
∴ By intermediate value theorem,
there has to be point ‘c’ between 1 and 2 such that f(c) = 0.
There is a root of the given equation between 1 and 2.
Question 17.
Let f(x) = ax + b (where a and b are unknown)
= x2 + 5 for x ∈ R
Find the values of a and b, so that f(x) is continuous at x = 1.
Solution:
f(x) = x2 + 5, x ∈ R
∴ f(1) = 1 + 5 = 6
If f(x) = ax + b is continuous at x = 1, then
f(1) = limx→1(ax+b) = a + b
∴ 6 = a + b where, a, b ∈ R
∴ There are infinitely many values of a and b.
Question 18.
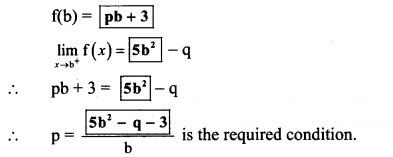
Activity: Suppose f(x) = px + 3 for a ≤ x ≤ b
= 5 x2 – q for b < x ≤ c
Find the condition on p, q, so that f(x) is continuous on [a, c], by filling in the boxes.
Solution: