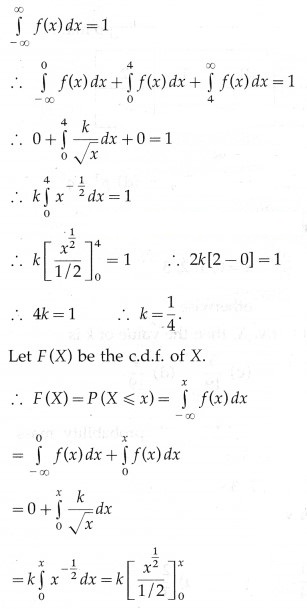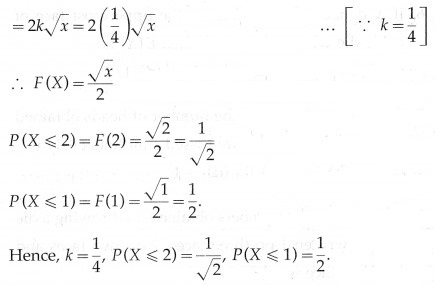(I) Choose the correct option from the given alternatives:
Question 1.
P.d.f. of a c.r.v. X is f(x) = 6x(1 – x), for 0 ≤ x ≤ 1 and = 0, otherwise (elsewhere) If P(X < a) = P(X > a), then a =
Question 3.

Question 5.
Question 7.
Question 8.
If the d.r.v. X has the following probability distribution:
Question 9.
If the d.r.v. X has the following probability distribution:
then k =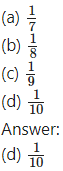
Question 10.
Find the expected value of X for the following p.m.f.
(a) 0.85
(b) -0.35
(c) 0.15
(d) -0.15
Answer:
(b) -0.35
(II) Solve the following:
Question 1.
Identify the random variable as either discrete or continuous in each of the following. If the random variable is discrete, list its possible values:
(i) An economist is interested in the number of unemployed graduates in the town of population 1 lakh.
(ii) Amount of syrup prescribed by a physician.
(iii) The person on a high protein diet is interesting to gain weight in a week.
(iv) 20 white rats are available for an experiment. Twelve rats are males. A scientist randomly selects 5 rats, the number of female rats selected on a specific day.
(v) A highway-safety group is interested in studying the speed (in km/hr) of a car at a checkpoint.
Solution:
(i) Let X = number of unemployed graduates in a town.
Since the population of the town is 1 lakh, X takes the finite values.
∴ random variable X is discrete.
Range = {0, 1, 2, …, 99999, 100000}.
(ii) Let X = amount of syrup prescribed by a physician.
Then X takes uncountable infinite values.
∴ random variable X is continuous.
(iii) Let X = gain of weight in a week
Then X takes uncountable infinite values
∴ random variable X is continuous.
(iv) Let X = number of female rats selected on a specific day.
Since the total number of rats is 20 which includes 12 males and 8 females, X takes the finite values.
∴ random variable X is discrete.
Range = {0, 1, 2, 3, 4, 5}
(v) Let X = speed of .the car in km/hr.
Then X takes uncountable infinite values
∴ random variable X is continuous.
Question 2.
The probability distribution of discrete r.v. X is as follows:
(i) Determine the value of k.
(ii) Find P(X ≤ 4), P(2 < X < 4), P(X ≥ 3).
Solution:

Question 3.
The following is the probability distribution of X:
Find the probability that
(i) X is positive
(ii) X is non-negative
(iii) X is odd
(iv) X is even.
Solution:
(i) P(X is positive) = P(X = 1) + P(X = 2) + P(X = 3)
= 0.25 + 0.15 + 0.1
= 0.50
(ii) P(X is non-negative)
= P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3)
= 0.20 + 0.25 + 0.15 + 0.1
= 0.70
(iii) P(X is odd)
= P(X = -3) + P(X = -1) + P(X = 1) + P(X = 3)
= 0.05 + 0.15 + 0.25 + 0.1
= 0.55
(iv) P(X is even)
= P(X = -2) + P(X = 0) + P(X = 2)
= 0.10 + 0.20 + 0.15
= 0.45.
Question 4.
= P(X = 5) + P(X = 4) + P(X = 3)
= P(X ≥ 3)
∴ P(X ≤ 2) = P(X ≥ 3).
Question 5.
In the p.m.f. of r.v. X
Find a and obtain c.d.f. of X.
Solution:
For p.m.f. of a r.v. X![]()
∴ P(X = 1) + P(X = 2) + P(X = 3) + P(X = 4) + P(X = 5) = 1
Let F(x) be the c.d.f. of X.
Then F(x) = P(X ≤ x)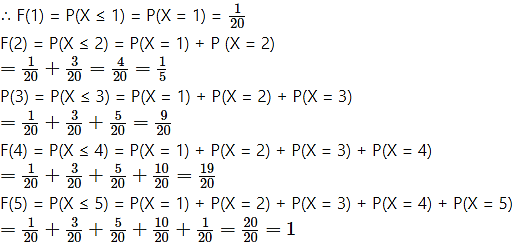
Hence, the c.d.f. of the random variable X is as follows:
Question 6.
A fair coin is tossed 4 times. Let X denote the number of heads obtained. Write down the probability distribution of X. Also, find the formula for p.m.f. of X.
Solution:
When a fair coin is tossed 4 times then the sample space is
S = {HHHH, HHHT, HHTH, HTHH, THHH, HHTT, HTHT, HTTH, THHT, THTH, TTHH, HTTT, THTT, TTHT, TTTH, TTTT}
∴ n(S) = 16
X denotes the number of heads.
∴ X can take the value 0, 1, 2, 3, 4
When X = 0, then X = {TTTT}
∴ n (X) = 1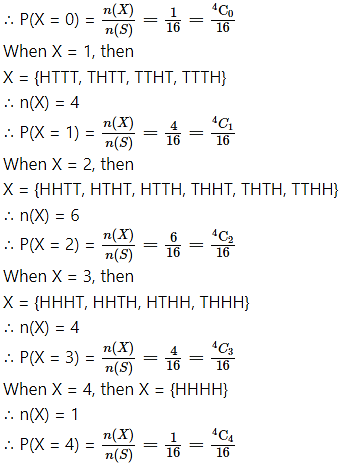
∴ the probability distribution of X is as follows: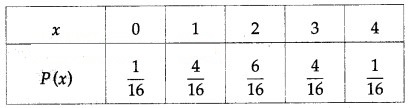
Also, the formula for p.m.f. of X is![]()
Question 7.
Find the probability distribution of the number of successes in two tosses of a die, where success is defined as
(i) number greater than 4
(ii) six appear on at least one die.
Solution:
When a die is tossed two times, we obtain (6 × 6) = 36 number of observations.
Let X be the random variable, which represents the number of successes.
Here, success refers to the number greater than 4.
P(X = 0) = P(number less than or equal to 4 on both the tosses)![]()
P(X = 1) = P(number less than or equal to 4 on first toss and greater than 4 on second toss) + P(number greater than 4 on first toss and less than or equal to 4 on second toss)
Thus, the probability distribution is as follows:
(ii) Here, success means six appears on at least one die.

Question 8.
A random variable X has the following probability distribution:
Determine:
(i) k
(ii) P(X > 6)
(iii) P(0 < X < 3).
Question 9.
The following is the c.d.f. of a r.v. X:
Find
(i) p.m.f. of X
(ii) P( -1 ≤ X ≤ 2)
(iii) P(X ≤ X > 0).
Solution:
(i) From the given table
F(-3) = 0.1, F(-2) = 0.3, F(-1) = 0.5
F(0) = 0.65, f(1) = 0.75, F(2) = 0.85
F(3) = 0.9, F(4) = 1
P(X = -3) = F(-3) = 0.1
P(X = -2) = F(-2) – F(-3) = 0.3 – 0.1 = 0.2
P(X = -1) = F(-1) – F(-2) = 0.5 – 0.3 = 0.2
P(X = 0) = F(0) – F(-1) = 0.65 – 0.5 = 0.15
P(X = 1) = F(1) – F(0) = 0.75 – 0.65 = 0.1
P(X = 2) = F(2) – F(1) = 0.85 – 0.75 = 0.1
P(X = 3) = F(3) – F(2) = 0.9 – 0.85 = 0.1
P(X = 4) = F(4) – F(3) = 1 – 0.9 = 0.1
∴ the p.m.f of X is as follows:
(ii) P(-1 ≤ X ≤ 2) = P(X = -1) + P(X = 0) + P(X = 1) + P(X = 2)
= 0.2 + 0.15 + 0.1 + 0.1
= 0.55
(iii) (X ≤ 3) ∩ (X > 0)
= { -3, -2, -1, 0, 1, 2, 3} n {1, 2, 3, 4}
= {1, 2, 3}
Question 10.
Find the expected value, variance, and standard deviation of the random variable whose p.m.f’s are given below:
Solution:
(i) We construct the following table to find the expected value, variance, and standard deviation:
(ii) We construct the following table to find the expected value, variance, and standard deviation:

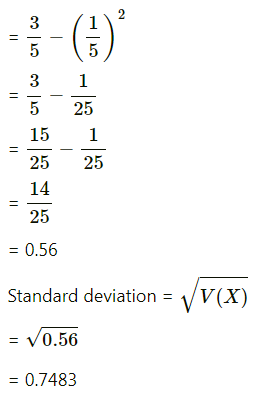
(iii) We construct the following table to find the expected value, variance, and standard deviation:


(iv) We construct the following table to find the expected value, variance, and standard deviation:

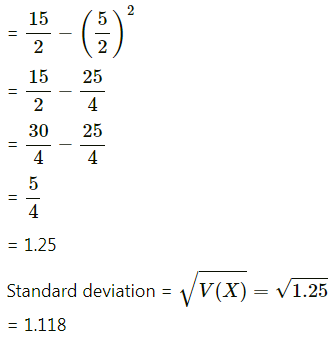
Question 11.
A player tosses two wins. He wins ₹ 10 if 2 heads appear, ₹ 5 if 1 head appears and ₹ 2 if no head appears. Find the expected winning amount and variance of the winning amount.
Solution:
When a coin is tossed twice, the sample space is
S = {HH, HT, TH, HH}
Let X denote the amount he wins.
Then X takes values 10, 5, 2.
We construct the following table to calculate the mean and the variance of X:

= 38.5 – 30.25
= 8.25
∴ Hence, expected winning amount = ₹ 5.5 and variance of winning amount = ₹ 8.25.
Question 12.
We construct the following table to calculate the mean and variance of X: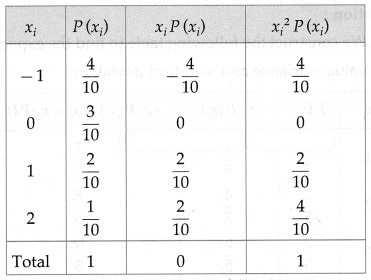
From the table
= 1 – 0
= 1
Hence, E(X) = 0, Var (X) = 1.
Question 13.
Suppose the error involved in making a certain measurement is a continuous r.v. X with p.d.f.
f(x) = k(4 – ), -2 ≤ x ≤ 2 and = 0 otherwise.
Compute
(i) P(X > 0)
(ii) P(-1 < X < 1)
(iii) P(X < -0.5 or X > 0.5).
Solution:
(i) P(X > 0)
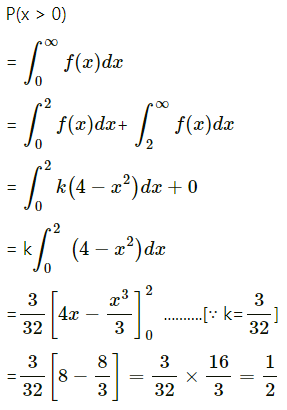
(ii) P(-1 < X < 1)

(iii) P(X < -0.5 or X > 0.5)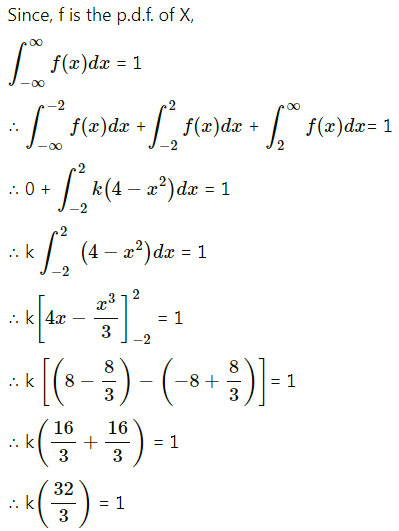
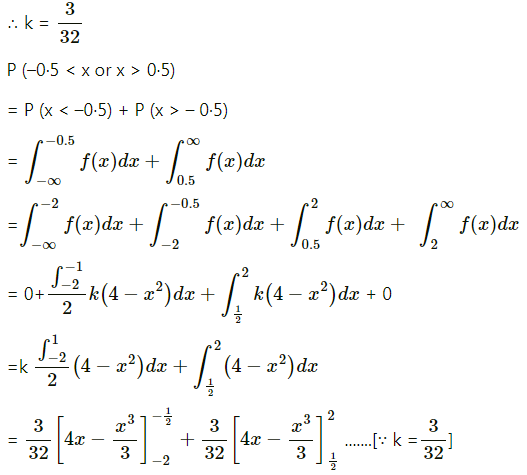


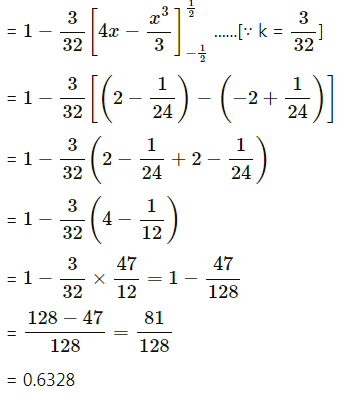
Question 14.
Solution:
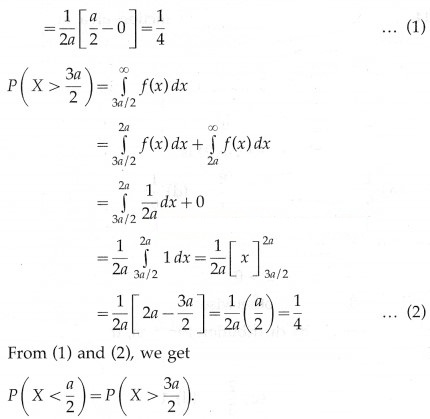
Question 15.
Solution:
Since f is p.d.f. of the r.v. X,